Environmental Project No. 1178, 2007
Evaluation of Pesticide Scenarios for the Registration Procedure
Contents
- 2.1. Overview of all scenarios
- 2.2. History and Description of Present Scenarios
- 2.3. History and Description of the PLAP Scenarios
- 2.4. Calibration and Validation
3. Scenario Comparison with Respect to Pesticide Leaching
- 3.1. Conditions for Scenarios
- 3.2. Comparison of Organic, Clay, and Silt Content in the Scenarios
- 3.3. Modelling Results
- 3.4. Summary and concluding remarks
4. Impact of Application Date on leaching
- 4.1. Conditions for Analysis
- 4.2. Modelled Balances and Leaching Results
- 4.3. Summary and Concluding Remarks
5. New Model Scenarios for the Registration Procedure of Pesticides
- 5.1. Selection of New Model Scenarios
- 5.2. Description of Themes included in the Standard of Reference
Preface
This study presents an evaluation of the two original model scenarios (Langvad and Karup) and the more recent EU-scenario (Hamburg) used by the Danish Environmental Protection Agency in their pesticide approval system. Based on the results two alternative scenarios are proposed representing respectively a sand and a clay soil in Denmark.
The study has been funded by the Danish Environmental Protection Agency. A steering group has been supervising the progress of project. The members of this group have been:
Jørn Kirkegaard, Danish Environmental Protection Agency (Danish EPA), Project owner.
Heidi C. Barlebo, Geological Survey of Denmark and Greenland (GEUS), Project leader.
Steen Marcher, Danish Environmental Protection Agency (Danish EPA).
Carsten Petersen, The Royal Veterinary and Agricultural University (KVL), Department of Agricultural Sciences.
Hans Jørgen Albrechtsen, Techinical University of Denmark (DTU), Institute of Environment and Resources.
Nick Jarvis, Swedish University of Agricultural Sceinces (SLU) Department of Soil Sciences, Division of Environmental Physics.
Ole Jensen, Syngenta Crop Protection A/S.
Heidi C. Barlebo
Copenhagen, April 2007
Sammenfatning
Miljøstyrelsen anvender resultater fra numeriske modeller i den danske godkendelsesordning for pesticider. Model-resultaterne stammer fra EU-scenariet Hamburg (sand) samt de danske scenarier Karup (sand) og Langvad (ler). I alle tre tilfælde er de data, der ligger til grund for scenarierne, meget sparsomme. Hamburg scenariet er ikke kalibreret, og Karup og Langvad scenarierne er kun blevet kalibreret mod en årlig vandbalance beregnet af en regional model. For nylig er der i projektet ”Varslingssystemet for udvaskning af pesticider til grundvandet (PLAP)” tilvejebragt et omfattende datasæt samt vel-parameteriserede og –kalibrerede modeller, der giver en god beskrivelse af vand- og stof-transport gennem den umættede zone på fem lokaliteter i Danmark. Tilvejebringelsen af de forbedrede datasæt har gjort det muligt at vurdere troværdigheden og repræsentativiteten af de tre scenarier, der p.t. benyttes i den danske godkendelsesordning.
Formålet med denne undersøgelse er at evaluere troværdigheden og repræsentativiteten af både de eksisterende model scenarier (Karup, Langvad og Hamburg) og de nyligt etablerede PLAP-scenarier (Jyndevad, Tylstrup, Silstrup, Estrup og Faardrup) med henblik på at anbefale to scenarier til anvendelse i den kommende godkendelsesprocedure.
Konklusionen er, at PLAP-scenarierne Jyndevad (sand) og Silstrup (ler) anbefales anvendt i den fremtidige danske godkendelseprocedure for pesticider. Anbefalingen er baseret på en karaterisering af nedbør og geologi samt kvalitet af feltdata og konceptuel model. Derudover er den baseret på forfatternes antagelse, at udvælgelse af det scenarie, der udvasker mest, bedst vil kunne beskytte det danske grundvand mod pesticider. Det skal dog bemærkes, at ingen af scenarierne præsenteret i denne rapport er blevet valideret mod pesticid udvaskningsdata. Mens de udvalgte PLAP-scenarier giver en god model beskrivelse af transporten af vand og konservativt stof, er deres prediktionsevne m.h.t. pesticid-udvaskning ikke blevet undersøgt. En sådan undersøgelse vil forøge troværdigheden af model-resultaterne betydeligt, og de nødvendige målte pesticid udvasknings data for PLAP-scenarierne er nu tilgængelige.
Denne rapport giver en grundig dokumentation af de udvalgte scenarier herunder resultater fra kalibrering mod målt grundvandsspejl, jordvands indhold, målte bromid koncentrationer og for lerjordene drænvandsafstrømning. Derudover er jordens tekstur (organisk indhold, ler og silt), simuleret vandbalance og simuleret udvaskning fra de nuværende scenarier sammenlignet med tilsvarende fra PLAP-scenarierne. Resultater fra sidstnævnte viser, at de nuværende scenarier (Karup, Langvad og Hamburg) er mindre restriktive m.h.t. udvaskning end PLAP-scenarierne. For sand scenarierne blev den største udvaskning fundet for Jyndevad. Den noget mindre udvaskning for Karup svarer til den fra Tylstrup, mens udvaskningen for Hamburg er betydelig mindre. For ler scenarierne gælder at udvaskningen for Langvad er større end for Faardrup, men betydeligt lavere end for Silstrup og Estrup. En undersøgelse af betydningen af udbringelsestidspunktet på simuleret pesticid udvaskning for PLAP-scenarierne viser, at valget af udbringelsestidspunkt og den dertilhørende planteudvikling kan have indflydelse på hvilke typer af pesticider, der kan komme igennem den danske godkendelsesordning for pesticider.
Summary
The Danish Environmental Protection Agency is currently using mathematical modelling results to support their pesticide approval procedure. Up to now the modelling results derives from the EU scenario Hamburg (sand) as well as the two Danish model scenarios Karup (sand) and Langvad (clay). The data supporting the three scenarios is very limited. Hamburg is thus not supported by any calibration and Karup and Langvad has been calibrated against a large scale yearly water balance only. Recently the Danish Pesticide Leaching assessment Programme (PLAP) has generated extensive data sets and well-parameterized and -calibrated model providing a good description of water and solute transport through unsaturated zone in Denmark. This improved data availability made it possibly to assess the reliability and representativeness of the scenarios hitherto being used in the Danish registration procedure.
The objective of this study was to evaluate the reliability and representativeness of both the existing model scenarios (Langvad, Karup and Hamburg) and the recent PLAP scenarios (Jyndevad, Tylstrup, Silstrup, Estrup and Faardrup), with the purpose of recommending the two scenarios to be used in the coming registration procedure.
The PLAP scenarios Jyndevad (sand) and Silstrup (clay) are proposed for future use in the Danish pesticide approval procedure. This recommendation is based on characterization with respect to precipitation, geology, reliability of field data and conceptual model. Additionally it is based on the authors´ assumption that choosing the worst case scenarios with respect to leaching will best protect the Danish groundwater against pesticides. However, it should be noted that none of the scenarios presented in this report have been validated against pesticide leaching data. While the selected PLAP scenarios provide a good model description of water and conservative solute transport its predicting capability towards pesticide leaching has not yet been assessed. Such assessment would attach further reliability to the model output, and the needed measured pesticide leaching data for the PLAP-scenarios is now available.
This report provides solid documentation on the selected scenarios including result from the calibration made against measured groundwater table, soil water contents, measurements of bromide concentration, and for the clay soils drainage runoff. Moreover soil texture (organic matter, clay, silt), simulated water balance, and simulated leaching from the existing scenarios are compared with those obtained from the PLAP scenarios. Results from the latter showed that the existing scenarios (Karup, Langvad, and Hamburg) were less restrictive with regard to leaching than the PLAP scenarios. For the sandy scenarios, the highest leaching was found at Jyndevad. The somewhat lower leaching at Karup resembles that of Tylstrup, while that of Hamburg was markedly lower. For clay scenarios, the leaching at Langvad was higher than that at Faardrup but considerably lower than that of Silstrup and Estrup. Furthermore, the choice of application date and plant evolution could influence which type of pesticides that will pass the Danish pesticide approval system.
1. Introduction
The Danish Environmental Protection Agency is currently using mathematical modelling results to support their pesticide approval procedure. Up to now the modelling results derives from the EU scenario Hamburg (sand) as well as the two Danish model scenarios Karup (sand) and Langvad (clay), see Appendix A, B and E for further details. The data supporting the three scenarios is very limited. Hamburg is thus not supported by any calibration, and Karup and Langvad have been calibrated against a large scale yearly water balance only.
However, the improved data availability obtained in recent years has made it possibly to assess the reliability and representativeness of the scenarios hitherto being used in the Danish registration procedure. Studies providing field data including/excluding model setup describing pesticides leaching in Denmark comprise:
- Field studies on plot scale of movement of herbicides and soil particles to field drainage tiles have been conducted at Højbakkegård, west of Copenhagen (Petersen et al., 2002).
- Large scale modelling project developing a numerical model for prediction of pesticides in surface water in the catchments of Lillebæk and Odder (Miljøstyrelsen, 2004).
- The Danish Pesticide Leaching Assessment Programme (PLAP), an intensive monitoring programme aimed at evaluating the leaching risk of pesticides under field conditions (Lindhardt et al., 2001; Kjær et al., 2004). Detailed pedological and hydro geological characterisation and an intensive long term monitoring enabled transport and leaching of applied pesticides and conservative tracers at five field sites.
Among the three studies, this project’s steering committee decided to make use of the PLAP data, which provide the most extensive data sets and well-parameterized and -calibrated model scenarios of flow and bromide transport.
The objective of this study was thus to:
- Present an overview of all eight scenarios with regard to the physical soil properties and the climatic conditions.
- Give a historical and site specific description of the present model scenarios (Langvad, Karup, and Hamburg) and the PLAP scenarios (Jyndevad, Tylstrup, Silstrup, Estrup, and Faardrup) including a short description of the numerical models behind the scenarios.
- Compare the simulated pesticide leaching ability of the present model scenarios’ with that of the PLAP-scenarios.
- Assess the impact of application date on the simulated pesticide leaching of the PLAP scenarios.
- Evaluate the reliability and representativeness of the present model scenarios and the PLAP scenarios with the purpose of recommending the two scenarios to be used in the coming registration procedure.
2. Presentation of Scenarios
- 2.1. Overview of all scenarios
- 2.2. History and Description of Present Scenarios
- 2.3. History and Description of the PLAP Scenarios
- 2.4. Calibration and Validation
The chapter presents an overview of yearly climatic conditions and central soil and hydraulic parameters of the eight scenarios used in the analysis: The two original model scenarios Langvad and Karup, the EU scenario Hamburg, and the five PLAP scenarios Tylstrup, Jyndevad, Silstrup, Estrup and Faardrup (Figure 1). Furthermore it states the stage of calibration/validation and gives the history and a more detailed description of each scenario.
Figure 1. Geographical location of field sites representing the not official EU-FOCUS-scenario Langvad and Karup, the EU-FOCUS-scenario Hamburg and the PLAP scenarios Tylstrup, Jyndevad, Silstrup, Estrup and Faardrup.
2.1. Overview of all scenarios
Organic matter, clay, and silt content in A, B, and C horizons for the sand and clay scenarios are illustrated in Figure 2. The values behind the figure added with other physical properties for the soils are given in Table1 and Table 2. The values are for most of the scenarios based on a more detailed data set, Appendix A, B, and C.
Organic matter, reaching 2.9% in sandy top soils and 4.1% in loamy topsoil, is decreasing with depth for all scenarios,
. The sandy scenarios have clay content less than 8% with small deviations for all horizons, and silt content with larger deviations ranging between 0.0-26.3%. At the clay scenarios, the horizons have 14% - 28% clay and 18% - 40% silt.
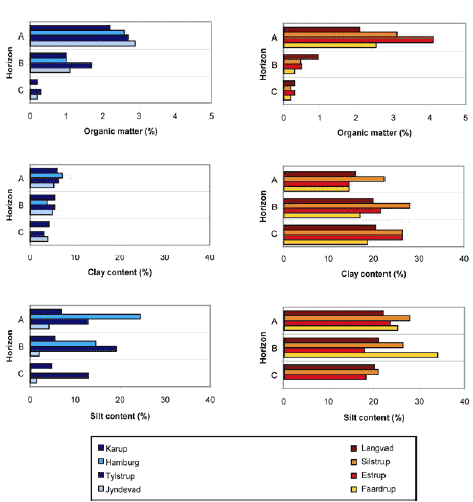
Figure 2. Organic matter, clay, and silt content in A, B, and C horizons for sand scenarios (left) and clay scenarios (right). The values of the PLAP-scenarios represent the average values used in the model set up.
An overview of climatic conditions for the eight scenarios is given in Figure 3, which shows maximum, minimum, and average yearly precipitation, actual evapotranspiration, and calculated net precipitation for each location for the studied 20-years period. Average precipitation varies between 650 and 1050 mm/year, with the lowest values for the two scenarios Langvad and Faardrup located in the eastern part of Denmark. Average actual evapotranspiration ranges between 400 – 550 mm/year, with the highest actual evapotranspiration at Jyndevad and hereafter Hamburg. Potential evapotranspiration is unfortunately not available for all scenarios, why the actual evapotranspiration that has been calculated for spring cereals in each scenario is shown in the figure. It is noted that the actual evapotranspiration of the clay scenarios (Langvad, Silstrup, Estrup, and Faardrup) calculated by MACRO have the same overall level, which is not the case for the sand scenarios (Karup, Tylstrup, and Jyndvad). Since geographical variation in the potential evapotranspiration is minimal (Kjær et al., 2005), this difference in simulated actual evapotranspiration for sand/clay scenarios seems to be related to the depth to and fluctuations of the groundwater table, and the root depth. The groundwater tables of the clay PLAP scenarios fluctuate generally between 1 and 4 m b.g.s. minimizing the root depth, whereas fluctuations of 1 metre are observed at the sand PLAP scenarios leaving the maximum level of the groundwater table, which is 3 and 1 m b.g.s. at respectively Tylstrup and Jyndevad, to be a controlling parameter. Yearly net precipitation (precipitation minus actual evapotranspiration) ranges between 25-1000 mm/year and average net precipitation varies between 250 mm/year at Faardrup and 650 mm/year at Estrup.
Table 1. Overview of physical properties for the sand profiles. For more detailed information see Appendix A, B, and C.
| Profile | Horizon | Depth (cm) |
Clay* (%) |
Silt* (%) |
Sand* (%) | OM (%) |
Bulk density (g/cm³) |
Porosity (%) |
Ks (m/s) |
| Karup | |||||||||
| A | 0-20 | 6.0 | 7.0 | 87.0 | 2.20 | 1.51 | 42 | 6.0e-5 | |
| B | 20-40 | 5.5 | 5.5 | 89.0 | 1.74 | 1.55 | 42 | 3.5e-5 | |
| B | 40-70 | 5.5 | 5.5 | 89.0 | 0.30 | 1.55 | 42 | 3.5e-5 | |
| C | 70- | 4.2 | 4.8 | 91.0 | 0.20 | 1.53 | 42 | 2.0e-4 | |
| Hamburg | |||||||||
| A | 0-30 | 7.2 | 24.5 | 68.3 | 2.6 | 1.50 | 39 | 2.3e-5 | |
| B | 30-60 | 6.7 | 26.3 | 67.0 | 1.7 | 1.60 | 37 | 3.2e-5 | |
| B | 60-75 | 0.9 | 2.9 | 96.2 | 0.3 | 1.56 | 35 | 2.8e-5 | |
| C | 75-200 | 0.0 | 0.1 | 99.9 | 0.0 | 1.61 | 31 | 2.8e-5 | |
| Tylstrup | |||||||||
| A | 0-32 | 6.4 | 12.9 | 80.7 | 2.7 | 1.40 | 47 | 7.0e-7 | |
| B | 32-93 | 5.5 | 19.2 | 75.3 | 1.7 | 1.42 | 47 | 5.0e-6 | |
| C | 93-160 | 3.1 | 13 | 83.9 | 0.3 | 1.51 | 43 | 2.5e-5 | |
| Jyndevad | |||||||||
| A | 0-31 | 5.3 | 4.2 | 90.5 | 2.9 | 1.40 | 47 | 3.5e-5 | |
| B | 31-95 | 5.0 | 2.0 | 93.0 | 1.1 | 1.45 | 45 | 1.3e-4 | |
| C | 95-150 | 4.0 | 1.5 | 94.5 | 0.2 | 1.50 | 43 | 2.5e-4 |
*For Tylstrup and Jyndevad: Clay:<2 μm; Silt: 2-50μm; Sand: 50–2000 μm. For Hamburg and Karup the intervals are not defined; OM: Organic matter determined as 1.72 · total organic carbon;
Ks: Saturated hydraulic conductivity. At Tylstrup and Jyndevad determined based on measurements for small soil cores (Iversen et al., 2001) from a couple of profiles. For Karup and Hamburg no information about sample size and number is available.
Table 2. Overview of physical properties for the clay profiles. For more detailed information see Appendix A and C.
| Profile | Horizon | Depth (cm) |
Clay* (%) |
Silt* (%) |
Sand* (%) | OM (%) |
Bulk density (g/cm³) |
Porosity (%) |
Ks (m/s) |
| Langvad | |||||||||
| A | 0-20 | 16.0 | 22.0 | 62.0 | 2.10 | 1.50 | 45 | 2.5e-5 | |
| B | 20-40 | 20.0 | 21.0 | 59.0 | 1.42 | 1.65 | 36 | 1.0e-4 | |
| B | 40-70 | 20.0 | 21.0 | 59.0 | 0.50 | 1.65 | 36 | 1.0e-4 | |
| C | 70- | 20.6 | 20.0 | 59.4 | 0.26 | 1.76 | 34 | 7.2e-6 | |
| Silstrup | |||||||||
| A | 0-30 | 22.5 | 27.7 | 49.8 | 3.1 | 1.48 | 44 | 3.5e-6 | |
| B | 30-95 | 27.9 | 26.2 | 45.9 | 0.5 | 1.61 | 40 | 2.4e-5 | |
| C | 95-190 | 26.3 | 20.9 | 52.8 | 0.2 | 1.75 | 34 | 2.0e-7 | |
| Estrup | |||||||||
| A | 0-27 | 14.6 | 23.3 | 62.1 | 4.1 | 1.51 | 43 | 3.5e-6 | |
| B | 27-55 | 21.7 | 17.9 | 60.4 | 0.5 | 1.68 | 36 | 8.0e-8 | |
| C | 55-150 | 26.3 | 18.2 | 55.5 | 0.3 | 1.64 | 38 | 3.0e-8 | |
| Faardrup | |||||||||
| A | 0-32 | 14.6 | 25.0 | 60.4 | 2.5 | 1.51 | 41 | 2.3e-6 | |
| B | 32-110 | 17.0 | 33.9 | 49.1 | 0.3 | 1.67 | 37 | 1.2e-5 | |
| C | 110-185 | 18.7 | 27.0 | 54.3 | 0.2 | 1.82 | 32 | 8.2e-7 |
*For Silstrup, Estrup and Faardrup: Clay:<2 μm; Silt:2-50μm; Sand: 50–2000 μm. For Langvad the intervals are not defined; OM: Organic matter determined as 1.72 · total organic carbon;
Ks: Saturated hydraulic conductivity. At Silstrup, Estrup, and Faardrup determined based on measurements for small soil cores (Iversen et al., 2001) from a couple of profiles. For Langvad no information about sample size and number is available.
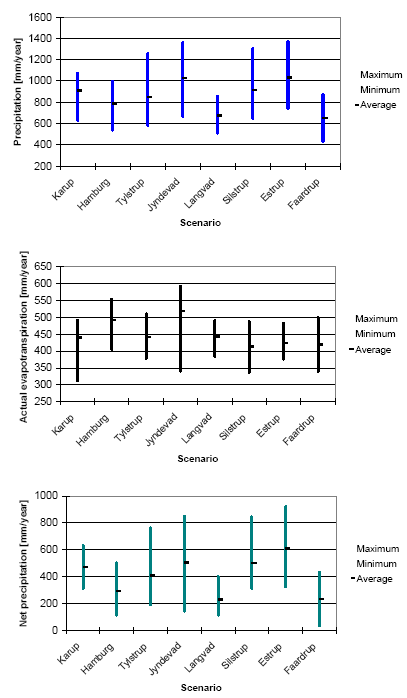
Figure 3. Maximum, minimum, and average yearly precipitation, actual evapotranspiration, and net precipitation for all locations for the 20-years period used in the simulations. Evapotranspiration is calculated for spring cereals. Net precipitation is calculated as precipitation minus actual evapotranspiration for spring cereals.
2.2. History and Description of Present Scenarios
2.2.1. Karup and Langvad Scenarios
The Karup and Langvad scenarios are the first model scenarios used in the Danish pesticide leaching approval system. They have been applied since 1998. The scenarios represent two typical Danish catchments investigated as a part of the Danish NPO-Research Programme (Storm et al., 1990), and reflect respectively the specific Western and Eastern type of combinations of soil types and climatic conditions found in Denmark. The two scenarios were originally set up in the numerical one-dimensional model MACRO 4.0 and later upgraded to MACRO 4.4.2. (FOCUS, 2000) and added in the FOCUS-setting without including them as FOCUS-scenarios. MACRO 4.4.2. contains the most advanced flow description of the model codes used in FOCUS as it includes preferential flow, but surface runoff and volatilisation are not represented. Additionally, MACRO 4.4.2. and most recent version MACRO 5.1. do not incorporate the possibility of simulating metabolite migration simultaneously with the parent compound making it less efficient and user friendly with regards to simulation of metabolite leaching.
The Karup scenario represents the Karup Å catchment located in West Jutland. Karup consists mainly of sandy soil types, and average precipitation and net recharge amount to 850 mm/y and 400 – 600 mm/y respectively, Figure 3 . The Langvad scenario represents the Langvad Å catchment located in East Sealand. The catchment consists of loamy moraine clay soils with topsoil of sandy loam, and the average precipitation and net recharge amount to 650 mm/y and 2000 mm/y respectively (Miljøstyrelsen, 1998). Yearly climatic conditions and soil and hydraulic properties for the two scenarios are summarised in Figure 2-3 and Table 2, whereas further information on data availability and model parameterisation is given in Appendix A. It is noted that precipitation and potential evaporation in the MACRO 4.4.2 setup of Karup and Langvad are only based on data from 1974 to 1993 and not from 1968 to 1996 as showed in Appendix A.
2.2.2. Hamburg Scenario
Many model runs have already been performed in the EU registration process. To facilitate the evaluation and minimise the model work especially with regard to metabolite calculations, the Danish EPA compared results from the nine FOCUS scenarios with results from the two Danish scenarios or at least with the Danish sandy scenario Karup. According to the Danish EPA this comparison showed quite similar results for Karup and the FOCUS Hamburg scenario. Given that the Hamburg scenario represents a German worst case groundwater scenario, and is geographically close to Denmark with comparable soil and climate data, the Danish EPA decided to accept modelling based on the Hamburg scenario (with the specified Danish input and output percentiles). The scenario is included in the group of FOCUS-scenarios and is setup in the numerical one- dimensional model code PELMO 3.2, which describes water flow in the soil in a rather simple manner (tipping bucket), but includes descriptions of surface runoff and volatilisation, and allows efficient and user friendly calculations for compounds with up to eight metabolites (FOCUS, 2000).
The Hamburg scenario is based on the national German scenario (reflecting soil properties and parameters at a field site situated at Borstel by Neustadt am Rübenberge), which is based on a soil survey intended to locate a worst case leaching soil. The scenario represents a sandy soil with an annual precipitation within the range of approximately 600 to 1000 mm/year. Vulnerability associated with this soil significantly exceeds the target of an 80th percentile soil (Kördel et al., 1989). An overview of yearly climatic conditions and soil and hydraulic properties for the scenario has been shown at the beginning of this chapter. Further information on data availability and model parameterisation is given in Appendix B.
2.3. History and Description of the PLAP Scenarios
In 1998, the Danish Parliament initiated the Pesticide Leaching Assessment Programme (PLAP). The objective of PLAP is to improve the scientific foundation for decision making in the Danish registration procedures for pesticides used in arable farming and hereby provide an early warning system for unacceptable leaching. The work was conducted by GEUS, the Danish Institute of Agricultural Sciences (DIAS), the National Environmental Research Institute (NERI) and the Danish Environmental Agency (EPA).
In 1998, no information was available concerning how to identify the most “vulnerable” soil types in Denmark as regards leaching of pesticides to the groundwater. For that reason, field sites were selected representing the dominant soil types and the climatic variation in Denmark. To ensure a short response time in the leaching of pesticides, the sites had shallow groundwater table located 1-4 m b.g.s.. Additional factors like hydrogeology, agricultural practice and site access were also taken into account.
Since July 1, 2003, the programme encompasses five sites, where continuously monitoring is performed from May 1999. A scenario for each site has been set up in the most recent version of MACRO, version 5.1.. This MACRO-version differs from the version used in FOCUS primarily by the numerical description of the soil water retention, the vertical discretisation and the lower boundary condition.
Of the five PLAP scenarios two (Tylstrup and Jyndevad) are located on sandy soil and three (Silstrup, Estrup, and Faardrup) on clayey soil (Figure 4). Moreover, Jyndevad, Silstrup and Estrup are situated in regions of Denmark with relative high precipitation, whereas Tylstrup and Faardrup represent regions with relative low precipitation. For further information, see Appendix C.
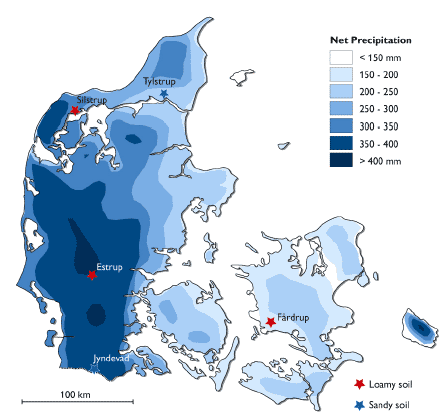
Figure 4. Location of the five PLAP sites (Tylstrup, Jyndevad, Silstrup, Estrup and Faardrup) in Denmark with map of yearly net precipitation. (The net precipitation in this figure is calculated as precipitation minus potential evaporation and therefore has lower values than shown in Figure 3).
2.4. Calibration and Validation
In the comparison of the scenarios it is important to keep in mind for each scenario their status of calibration and validation. This can be grouped with respect to the scenarios as follows:
- Karup and Langvad: Calibrated against a large scale yearly water balance. Some input parameters have been collected.
- Hamburg: An artificial scenario based on the National German Scenario. Some input parameters have been collected.
- Tylstrup, Jyndevad, Silstrup, Estrup, and Faardrup: The PLAP-model-scenarios have been: (1) setup based on an extensive amount of direct measurements of hydraulic and geological parameters; (2) calibrated using measured groundwater table, soil water content at three depths, drainage runoff at clay soils, bromide concentrations in suction cups, and bromide concentrations in drains for the period May 1999 – May 2004; and (3) validated against water balance and bromide transport for a two year period (Kjær et al., 2007). The results of the validation demonstrate, the PLAP-model-scenarios ability to predict percolation and bromide leaching,
It should be emphasised that none of these scenarios have been calibrated nor validated against direct measured pesticide transport. This is presently possible to do for the PLAP scenarios but is not a part of this study.
3. Scenario Comparison with Respect to Pesticide Leaching
- 3.1. Conditions for Scenarios
- 3.2. Comparison of Organic, Clay, and Silt Content in the Scenarios
- 3.3. Modelling Results
- 3.4. Summary and concluding remarks
In this chapter the eight model scenarios (Karup, Langvad, Hamburg, Tylstrup, Jyndevad, Silstrup, Estrup and Faardrup) are compared with respect to water and pesticide balance for the hole soil profile as well as pesticide leaching 1 and 3 m b.g.s..
3.1. Conditions for Scenarios
To enable comparison among the different model scenarios, crop parameters, dose, application time of pesticides, and parameters on model solutes are kept the same in all scenarios. Furthermore, it is assured that pesticide leaching of the PLAP scenarios is estimated in the same manner as in the not official EU-FOCUS-scenarios Langvad and Karup.
3.1.1. Crops
Crops comprised spring cereals and winter cereals. These crops are chosen to achieve compatibility of and consistency in the crop data for the scenarios, and to make the scenarios as representative as possible for any future use in the approval procedure of pesticides. The scenarios are set up having either spring cereals or winter cereals every year. Important dates for the crops are shown in Table 3.
Table 3. Important dates for crops used in the evaluation of scenarios.
| Crop | Emergence | Harvest |
| Spring cereals | April 1 | August 20 |
| Winter cereals | November 5 | August 10 |
The chosen crop-data for the spring and winter cereals of all the scenarios correspond with those used in the FOCUS-Hamburg-scenario (PELMO 3.3.2.), Figure 5. The crop-data used for the Langvad and Karup scenario (MACRO 4.4.2.), which are setup up in connection with the FOCUS-scenarios, is not available. Thus, it is assumed that similar crop-data for the spring and winter cereals is used in the Langvad and Karup scenario. For the PLAP-scenarios, the used crop-parameters are presented in Table 4. The cropping dates given in this table by Julian Day Number (JDN) represent the cropping dates presented in Figure 5, and the crop-properties resemble the spring barley and winter wheat used in a PLAP-calibration.
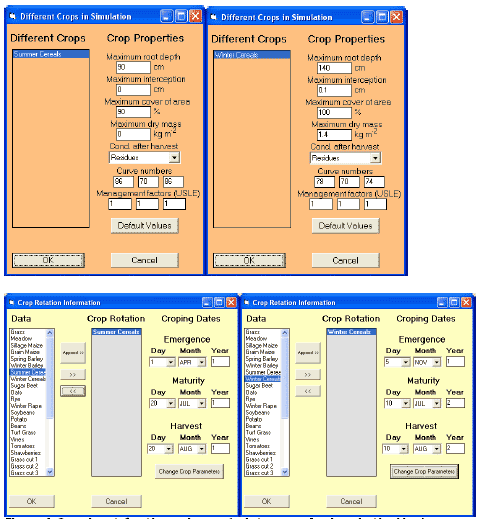
Figure 5. Crop-input for the spring and winter cereals given in the Hamburg-scenario´s PELMO 3.3.2.-setting.
Table 4. MACRO crop input parameters for the PLAP-scenarios. JDN: Julian Day Number where the days are counted starting from January 1 (i.e. January 1 equals JDN= 1 and January 2 equals JDN= 2).
| Crop properties | Spring cereals | Winter cereals |
| Day of crop emergence (IDSTART) | JDN = 90 (April 1) | JDN = 300 (November 5) |
| Day of Intermediate crop development stage (ZDATEMIN) | JDN = 91 | JDN = 66 |
| Day of maximum leaf area/root depth (IDMAX) | JDN = 201 | JDN = 191 |
| Day of harvest (IHARV) | JDN = 232 | JDN = 222 |
| Form factor controlling the rate of increase of leaf area between emergence and maximum leaf area (CFORM) | 2 | 2 |
| Form factor controlling the rate of decrease of leaf area between the date of maximum leaf area and harvest (DFORM) | 0.3 | 0.2 |
| Root distribution (RPIN) | 60% | 60% |
| Fraction pf the available water exhausted before reduction in transpiration occurs (FAWC) | 0.35 | 0.2 |
| Critical soil air content for root water uptake (CRITAIR) | 5% | 5% |
| Root adaptability factor | 0.2 | 0.1 |
| Canopy interception capacity (CANCAP) | 2mm | 3mm |
| Correction factor for wet canopy evaporation (ZALP) | 1 | 1 |
| Root depth at ZDATEMIN | 0.01m | 0.2m |
| Maximum root depth | 0.7m for clayey sites 0.6 m for sandy sites |
0.9m for clayey sites 0.6m for sandy sites |
| Leaf Area Index at ZDA TEMIN | 0.01 | 1 |
| Maximum Leaf Area Index | 4 | 6 |
| Leaf Area at harvest | 2 | 2 |
3.1.2. Dose and Application Dates
Pesticide is always applied in a dose of 1 kg/ha (100 mg/m²) every year over a period of 20 years + 6 years “warm-up” for the scenarios. This is incorporated for both the spring and fall application in all the scenarios. The dates of pesticides application for all the scenarios are assessed from crop specific emergence dates given in PELMO 3.3.2. together with management information from the PLAP-program providing information on the typical time period between emergence and application (Table 5).
Table 4. Crops, dose, emergence and application dates. The resembling Julian Day Number of the latter is given in brackets. JDN: seeTbale 4
| Crop | Dose | Emergence dates | Application date |
| Spring cereals | 1 kg/ha | April 1 | April 23 (113) |
| Winter cereals | 1 kg/ha | November 5 | November 20 (315) |
The application date is not adjusted to occurrence of precipitation, since daily precipitation data for the Hamburg, Langvad, and Karup scenarios are not available. MACRO in connection with the PLAP-scenarios can not directly simulate soil incorporation of plant protection products, why a minimal amount of irrigating water is required during application. As suggested in FOCUS (2000) 0.1 mm irrigation is applied. This application is chosen to have duration of 20 min based on experience from PLAP applications. The pesticide concentration in the irrigation water was 1·106 mg/m3 equalizing an application rate 100 mg/m². The fraction of irrigation intercepted by crop canopy is set to zero.
3.1.3. Model Solutes
The selection of model solutes A, B, and C has aimed at representing the range of the pesticide parameters characterising commonly used pesticides in European agriculture and at the same time representing pesticides, which are forbidden, percolation is registered, and the properties (DT50 and Koc) are well documented. The final choice of pesticides for representing the model solute is based on the pesticides ability to percolate through the upper meter in all the scenarios. The model solutes and their properties are presented in
Table 5.
For the PLAP-scenarios, the DT50 values in
Table 5 are representing the degradation in liquid and solid phase respectively in the macro- and micro-pore-regime in the upper approximately 30 cm b.g.s.. Below this depth, a depth factor on degradation is included. The DT50-values are divided by: 0.5 at app. 30-60 cm b.g.s. and 0.3 at app. 60-100 cm b.g.s. Below 1 meter it is assumed that no degradation takes place. This depth-related DT50-distribution is consistent with the DT50-distribution used within FOCUS, which include the Hamburg, Langvad and Karup scenarios.
Table 5. Properties for the model solutes: Pesticide A, B and C. Values were obtained from Linders et al., 1994; Roberts, 1998; Tomlin, 1997; Working documents from the European Commission.
| Pesticide | DT50 [days] |
Koc [ml/g] |
n | Vp [Pa] |
M [g/mol] |
S [mg/l] |
| A | 49 | 99.5 | 1.0 | 3.85E-5 | 216 | 33 |
| B | 6.1 | 30 | 0.99 | 2.3E-4 | 214.65 | 250 |
| C | 80 | 400 | 0.90 | 5.6E-5 | 342.2 | 100 |
3.1.4. Calculation of Leaching Output
Comparison between scenarios is based upon the yearly average pesticide concentration in leachate at 1 m b.g.s., which is not to exceed the maximum allowed concentration of 0.1 μg/l.. 1 m b.g.s. is the reference for decisions on pesticide approval when applying mathematical models and the background for this reference is explained in Appendix E.
For the scenarios setup in MACRO (Karup, Langvad, Tylstrup, Jyndevad, Silstrup, Estrup, and Faardrup), the value is calculated as the total annual leaching loss divided by the total annual flow. For the PLAP scenarios also the yearly average pesticide concentration in leachate at 3 m b.g.s. is calculated in this way.
For the Hamburg scenario setup in PELMO, it is not stated how the yearly average pesticide concentration in leachate at 1 m b.g.s. is calculated.
For the drained clay scenarios (Langvad, Silstrup, Estrup, and Faardrup), the yearly average pesticide concentration in leachate at 1 m b.g.s. may differ from the yearly average pesticide concentration in the drains, which are situated just below 1 metres depth. In MACRO, flux rates from macropore-saturated layers above the drainage depth contribute directly to drain flow based on the assumption that the drains are overlain by fully penetrating seepage surfaces (i.e. ditches or drains with highly permeable backfill). Unlike the leachate concentration, the yearly average pesticide concentration in the drains thus could include by-pass pesticide-flux from layers above the drain.
3.2. Comparison of Organic, Clay, and Silt Content in the Scenarios
Organic matter together with clay and silt content in the soil profile has been proven to have a large influence on pesticide leaching (GEUS, 2004). Therefore, these contents are summarized for the A, B, and C horizons for the sand and clay scenarios in Figure 2.
The content of organic matter, clay, and silt at Karup is within the range of content measured at the other sand sites though the organic matter in the A horizon is a little less than for the others. This is not the case for the Hamburg scenario, which is different from the Danish scenarios by not containing organic matter in the C horizon, containing much more silt in the A horizon and not containing clay or silt below the B horizon.
The Langvad scenario is characterized by having less organic matter in the A horizon and more organic matter in the B horizon than the other clay scenarios. All other contents of organic matter, clay, and silt are within the range of content measured at the other clay locations.
It is to be noted that the organic content in the B horizon generally is larger for the sand than the clay scenarios. The amount of organic matter found in the A horizon at Estrup should also be noticed.
3.3. Modelling Results
The results derived from 48 simulations representing the eight scenarios, two crops, and three pesticides (A, B, and C) will be presented in the following subparagraphs: Water balance, Mass balance, and Pesticide leaching. Results on an annual basis are to be found in Appendix D:
- For the Langvad and Karup scenario, yearly average flux concentrations in leachate at 1 metres depth, and mass balance diagrams including yearly dissipated, crop uptake, lost in runoff, and leached are presented.
- For Hamburg, yearly average flux concentrations in leachate at 1 metres depth and bottom of soil column, and mass balances including volatilisation, plant uptake, degradation, runoff, and percolate are presented.
- For the PLAP-scenarios, yearly average flux concentrations in leachate at 1 and 3 metres depth, and yearly water and mass balances including respectively: a) Precipitation+Irrigation, Actual Evaporation, Runoff, Percolate, and Drainage, and b) Leaching, Runoff, Degradation, Plant uptake, Storage+solute infiltration, and Drainage, are presented.
3.3.1. Water Balance
Obviously the driving variable precipitation has a great effect on pesticide leaching. Together with the calculated values for actual evapotranspiration, runoff, and drainage it gives the amount of percolation, which is decisive for leaching.
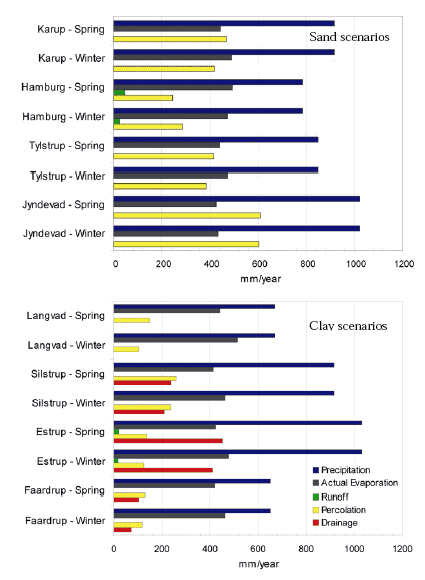
Figure 6. Water balance of 20 years simulation for all sand (upper) and clay (lower) scenarios including both spring and winter cereals. Information on an annual basis can be found in Appendix D. OBS: it was not possible to obtain drainage values for Langvad.
The water balances of the sand scenarios show that the balance values for Karup are in between the values for Tylstrup and Jyndevad, Figure 6. Hamburg differs from all others by having the least precipitation and percolation, and at the same time surface runoff. For Hamburg percolation is only about a third of the precipitation whereas for the other scenarios it is about half of the precipitation. This lower percolation at Hamburg could be a result of the water contribution to surface runoff, which is not present at the other sand scenarios. Surface runoff on sandy soils are seldom seen and can not be verified by the information found related to the Hamburg scenario. The presence of surface runoff at Hamburg could also be caused by the fact that Hamburg is set up in PELMO including a simple hydraulic description, whereas Karup, Tylstrup, and Jyndevad are set up in MACRO including a more theoretical correct advanced hydraulic description.
The water balances of the clay scenarios show that the values of the Langvad scenario resemble those of the Faardrup scenario, though keeping in mind drainage values are not available for Langvad, Figure 6. Due to the influence of especially the drainage system there is no clear correlation between total amount of precipitation and total amount of percolation. It is noticeable that Silstrup has almost twice as much percolation than the other clay scenarios, and Estrup has the largest amount of drainage and includes a minimal amount of surface runoff.
Looking at the water balance for all scenarios not surprisingly percolation is largest for the sand scenarios. The results also show that except for the Hamburg scenario, a winter crop results in a larger actual evapotranspiration. The actual evapotranspiration only varies between 400 and 550 mm/year for all scenarios while precipitation varies between 650 and 1050 mm/year (ref. chapter 2.1).
3.3.2. Mass Balance
In the understanding of leaching of a pesticide, it is crucial to follow the fate of the pesticide in the soil. In this study, it has been possible to distinguish between amount of pesticide transported to the drains, stored or infiltrated in the soil, removed by plant uptake, degraded, transported through runoff, or leached. This pesticide balance of the 20-years period for the whole profile is summarized for sand scenarios in Figure 7, while information on an annual basis can be found in Appendix D.
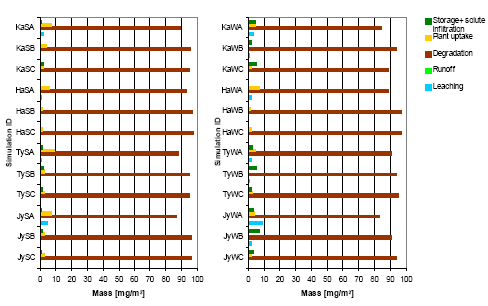
Figure 7. Mass balance of the 20-years period for the whole profile of the sand scenarios with spring (left) and winter (right) cereals. Example of simulation ID “KaSC”: Ka represents Karup, S represents spring application, and C represents pesticide C.
Before studying the balances in more detail, it is important to draw attention to the fact that the parameter controlling plant uptake in MACRO is a calibration parameter. For the PLAP scenarios the parameter, FSTAR, is 0.4 for Silstrup, 0.5 for Tylstrup, Jyndevad, and Faardrup, and 0.6 for Estrup. The parameter value for Karup, Langvad, and Hamburg is unknown or not included in the model setup.
Not surprisingly, the pesticide balance Figure 7-8 shows that between 64 and 97 percent of the applied pesticide in the scenarios are being degraded (64 to 97 mg/m²). Additionally, the plant uptake values are within 0.24-9 percent of the applied pesticide and are generally larger for the spring application than the fall application. It is noted that leaching and drainage are larger for fall applications than for spring applications, which is consistent with the larger amount of precipitation during fall and winter. Leaching is largest for pesticide A, which is moderately mobile and having a moderate half-life compared to Pesticide B and C. Pesticide B is most mobile but has the shortest half-life. Pesticide C is least mobile but has the largest half-life. Most leaching is simulated for Silstrup and second most for Jyndevad. Hardly any leaching is obtained for Faardrup.
The results for sand scenarios Figure 7 show that the estimated balance values for Karup for both spring and fall applications are in between the ones for Tylstrup and Jyndevad with Jyndevad leaching the most and Tylstrup the least. Hamburg resembles Tylstrup but does not have a PELMO-output resembling ‘storage + solute infiltration’, and does not result in leaching of Pesticide B for the fall application.
The results for clay scenarios Figure 8 show that Langvad has the largest plant uptake. Only for Estrup and Silstrup, the pesticides are transported to the drains but as stated previously, this information is not available for Langvad. At Langvad, leaching only occurs for fall application of Pesticide A and B and in very small concentrations. Thus with respect to leaching, Langvad resembles Estrup with Silstrup having much more leaching and Faardrup having hardly any leaching.
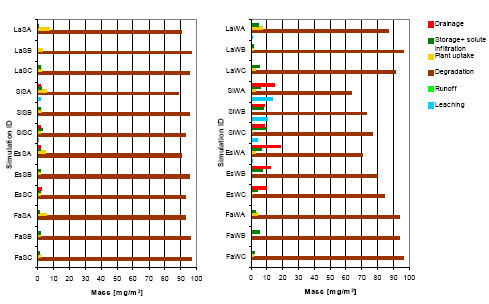
Figure 8. Mass balance of the 20-years period for the whole profile of clay scenarios with spring (left) and winter (right) cereals. The balance includes drainage, storage + solute infiltration, plant uptake, degradation, runoff, and leaching. Example of simulation ID “LaSC”: La represents Langvad, S represents spring application, and C represents pesticide C. OBS: it was not possible to obtain drainage values for Langvad.
3.3.3. Pesticide Leaching
Simulated average annual pesticide leaching at 1 m b.g.s. for sand and clay scenarios for the 20-years period is summarized in Figure 9–10. Additional information on an annual basis can be found in Appendix D. Note that the drain depth for the clay scenarios is below 1 m b.g.s.
Because MACRO is a 1-dimensional model, calculated concentrations below the groundwater table are questionable given the exclusion of the horizontal flow and transport component. In the vadose zone of the soil profile, the vertical flow and transport component will be dominating, thus the estimation of concentration with a 1-dimensional model would be acceptable. Nevertheless, the Danish EPA has a strong wish to see calculated concentrations at 3 m b.g.s., which often is below the groundwater table in the scenarios. Therefore, pesticide leaching at 3 m b.g.s. is provided for the PLAP scenarios in Figure 9-10. It was not possible to obtain this information for the other scenarios.
Results for all scenarios show that pesticide A is leaching in highest concentrations in both 1 and 3 m b.g.s. (only PLAP scenarios) for both spring and fall applications. Thus, all scenarios seem most vulnerable to the pesticide being moderately mobile and having a moderate half-life compared to the other chosen pesticides (Pesticide B is most mobile but has the shortest half-life. Pesticide C is least mobile but has the largest half-life). For spring application at Jyndevad, Langvad, Silstrup, and Estrup, pesticide C is leaching more than pesticide B. For all other spring applications it is the opposite. For fall applications, pesticide B is always leaching more than pesticide C.
As compared to spring application the fall application is resulting in a larger pesticide concentration in both 1 m and 3 m b.g.s. (only PLAP scenarios) for all three pesticides and eight scenarios. This is not surprising considering the larger precipitation during fall and winter. Moreover, residence time of the pesticides is generally shorter during fall due to larger amount of percolation occurring within the first months after application (Kjær et al., 2004). 34 of the 48 combinations of scenario, application time and pesticide have a calculated pesticide leaching above the maximum allowed concentration (MAC) equal to 0.1 μg/l.
![Figure 9. Average annual pesticide leachate at 1 and 3 m b.g.s. for sand scenarios with spring (left) and fall (right) application. Example of simulation ID “KaSC”: Ka represents Karup, S represents spring application, and C represents pesticide C. MAC is the maximum allowed concentration 0.1μg/l. The red numbers in brackets e.g. [18/20] represents the number of years out of the 20-years period (black number), where an annual pesticide concentration is equal to or beyond 0.1μg/l. Note that pesticide leaching at 3 m b.g.s. is not available for Karup and Hamburg.](images/fig09.gif)
Figure 9. Average annual pesticide leachate at 1 and 3 m b.g.s. for sand scenarios with spring (left) and fall (right) application. Example of simulation ID “KaSC”: Ka represents Karup, S represents spring application, and C represents pesticide C. MAC is the maximum allowed concentration 0.1μg/l. The red numbers in brackets e.g. [18/20] represents the number of years out of the 20-years period (black number), where an annual pesticide concentration is equal to or beyond 0.1μg/l. Note that pesticide leaching at 3 m b.g.s. is not available for Karup and Hamburg.
On sandy soils, leaching concentrations 1 m b.g.s. at Karup were found to resemble most the values obtained at Tylstrup. An exception is the smaller leaching concentration at 1 m b.g.s. for Pesticide B for fall application. Hamburg differs by generally having a lower leaching concentration, while that at Jyndevad was the highest.
On clayey soils, the leaching concentrations at 1 m b.g.s. at Langvad are higher than at Faardrup but considerably lower than Silstrup and Estrup. Silstrup generally has the highest leaching concentrations. Among the clay scenarios, Silstrup is characterized by having higher clay content and the largest calculated percolation, Figure 2 and Figure 6.
![Figure 10. Average annual pesticide leachate at 1 and 3 m b.g.s. for clay scenarios with spring (left) and fall (right) application. Example of simulation ID “LaSC”: La represents Langvad, S represents spring application, and C represents pesticide C. MAC is the maximum allowed concentration 0.1μg/l. [18/20] represents the fraction of years out of the 20-years period, where an annual pesticide concentration is equal to or beyond 0.1μg/l. Note that pesticide leaching at 3 m b.g.s. is not available for Langvad.](images/fig10.gif)
Figure 10. Average annual pesticide leachate at 1 and 3 m b.g.s. for clay scenarios with spring (left) and fall (right) application. Example of simulation ID “LaSC”: La represents Langvad, S represents spring application, and C represents pesticide C. MAC is the maximum allowed concentration 0.1μg/l. [18/20] represents the fraction of years out of the 20-years period, where an annual pesticide concentration is equal to or beyond 0.1μg/l. Note that pesticide leaching at 3 m b.g.s. is not available for Langvad.
3.4. Summary and concluding remarks
For the sand scenarios, the worst case scenario in relation to pesticide leaching is the Jyndevad scenario. Both the leaching part in the mass balance (Figure 7) and the pesticide concentrations in 1 and 3 m b.g.s. (Figure 9) for this scenario represent the maximum value of the four sand scenarios. The Jyndevad scenario is characterised by having the largest average and maximum net precipitation (Figure 3), the lowest clay and silt content in the A horizon, and the highest organic matter content in the A horizon (Figure 2) of the four sand scenarios. For the sand scenarios in general, the pesticide concentration 1 m b.g.s. is reduced going from applying Pesticide A to B to C, where C has the highest Koc value of the three pesticides (Table 6). This trend is not the case for Jyndevad with spring application; here the pesticide concentration 1 m b.g.s. is reduced going from applying Pesticide A to C to B. This could emphasis, the seasonal net precipitation impact on leaching ability of different pesticides. In connection with the spring application at Jyndevad, less percolation compared to the fall application could result in a larger amount of Pesticide B to decompose before reaching 1 metres depth (DT50=6.1, Table 6), therefore the DT50-value seems to play a larger role given the choice of the Koc–value in the pesticide B leaching.
Apart from the sand scenario Jyndevad representing worst case of leaching and Hamburg representing generally the lowest leaching, the pesticide concentrations 1 m b.g.s. for Karup and Tylstrup are quite similar even though the scenarios’ net precipitation and soil texture-setup vary, Figure 2-3.
For the clay scenarios, the worst case scenario in relation to pesticide leaching is the Silstrup scenario. Both the leaching and drainage part of the mass balance (Figure 8) and the pesticide concentration 1 and 3 m b.g.s. (Figure 9) for this scenario represent approximately, the maximum value of the four clay scenarios. The Silstrup scenario is characterised by having the second largest average and maximum net precipitation (Figure 3), the highest clay and silt content in the A horizon, and the second highest organic matter content in the A horizon (Figure 2) of the four clay scenarios. For the clay scenarios with fall application (Figure 10), the pesticide concentration 1 m b.g.s. is reduced going from applying Pesticide A to B to C like the general trend in the sand scenarios. This trend is though not the case for the spring applications; here the pesticide concentration in 1 m b.g.s. is reduced going from applying Pesticide A to C to B. This again seems to emphasis, the seasonal net precipitation impact on different pesticides’ leaching ability. Decomposition of the pesticide seems to play a larger role than sorption in the spring setting compared to the fall setting of the clay scenarios.
The pesticide concentrations 1 m b.g.s. for Langvad, Estrup and Faardrup are quite different. By ranking the scenarios after the one with the highest pesticide leaching including the drainage part, Figure 8, the following picture will appear: Estrup, Langvad, and Faardrup. Even though Langvad and Faardrup have a similar average net precipitation noticeable with larger variations in Faardrup (Figure 3), Langvad has larger pesticide leaching. Looking at the physical properties of the clay profiles (Figure 2 and Table 2), Faardrup has generally higher silt content and lower saturated hydraulic conductivity than Langvad, but to explain the difference in pesticide leaching ability between the scenarios more information concerning the drainage component at Langvad is needed.
4. Impact of Application Date on leaching
- 4.1. Conditions for Analysis
- 4.2. Modelled Balances and Leaching Results
- 4.3. Summary and Concluding Remarks
Many parameters can be the focus of a sensitivity analysis regarding pesticide leaching (Dubus and Brown, 2002; www.eu-footprint.org). In this chapter we focus on the date of application, since changing this date is a part of the registration procedure, Appendix E. For the five PLAP-scenarios, changes in pesticide A, B, and C leaching due to a change in application date including changes in plant evolution are studied. The purpose of this study is to examine if the choice of application date and plant evolution influence, which type of pesticides that will pass the Danish pesticide approval system.
4.1. Conditions for Analysis
For the five PLAP scenarios Tylstrup, Jyndevad, Silstrup, Estrup, and Faardrup, the dates for spring and fall application are changed to respectively about 2½ weeks later and a month earlier than the dates used in the previous chapter. Both new dates are realistic when looking at the agricultural practice in Denmark and are given in Table 6. For the fall application, the date used in the sensitivity analysis can be regarded as less conservative with respect to pesticide leaching than the date used in the evaluation of scenarios.
Table 6. Crops, dose, and application/emergence dates used annually in the sensitivity analysis and in the evaluation of scenarios (Chapter 4).
| Crop | Dose | Application/Emergence date used in sensitivity analysis |
Application/Emergence date used in evaluation of scenarios |
| Spring cereals | 1 kg/ha | May 10 / April 15 | April 23 / April 1 |
| Winter cereals | 1 kg/ha | October 20 / October 5 | November 20 /November 5 |
To obtain a realistic agreement between application date and plant evolution in the sensitivity analysis, the emergence date of respectively the spring and winter cereals are set to April 15 and October 5 instead of April 1 and November 5 in the evaluation of scenarios, Table 6. The sensitivity analysis is conducted for pesticides A, B, and C defined in Chapter 3.1.3.
4.2. Modelled Balances and Leaching Results
4.2.1. Water and Mass Balance
Generally, the water and mass balance of the whole profile at the PLAP scenarios were not very sensitive to the change in application date and plant evolution.
Changes in the water balance of the whole soil profile were only observed for Tylstrup with spring cereals and Estrup with both spring and winter cereals. By the change in application date and plant evolution:
§ the actual evaporation decreased with 6-10 mm/year for the three scenarios.
§ the percolation increased with 1-6 mm/year for the two scenarios with spring cereals.
§ the drainage at Estrup increased with 8 mm/year for the scenario with spring cereals and decreased with 7 mm/year for the scenario with winter cereals.
Changes in the mass balance of the whole soil profile for:
§ the sand scenarios were less than 2% for the leaching, runoff, degradation, plant uptake and storage + solute infiltration component.
§ the clay scenarios differs with up to 9%. Generally, the changed application date had minor effect on the runoff and plant uptake component, whereas:
o the scenarios with spring cereals generally gave rise to a decrease in leaching and storage + solute infiltration added with an increasing degradation and drainage,
o the scenarios with winter cereals generally gave rise to a decrease in leaching and degradation added with an increasing storage + solute infiltration and drainage.
4.2.2. Pesticide Leaching
Changing the application date of pesticides by ~3 weeks had following impact on estimated leaching concentration 1 m b.g.s.:
- At sandy soils only a minor increase in leaching from pesticide A and C was observed. A drastic increase in Pesticide B leaching was however observed at both spring and fall application at Tylstrup and at the fall application at Jyndevad, Figure 11
- At the clay soils only minor effect on leaching was observed. Generally the leaching increased slightly, exception being pesticide B and C applied on Estrup and pesticide A applied on Faardrup, all of which leaching were found to decrease.
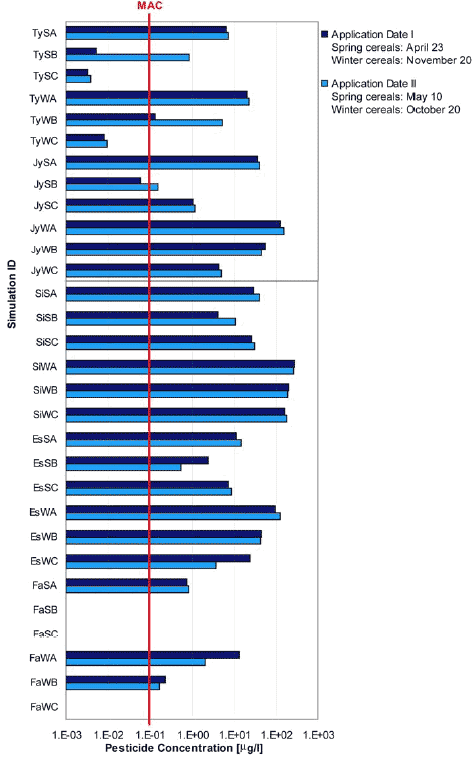
Figure 11. The yearly average pesticide leachate 1 m b.g.s. of a 20 years period respectively for Application Date I and II. The pesticide leachate 1 m b.g.s. is presented for sand and clay PLAP-scenarios with spring or winter cereals. Example of simulation ID “KaSC”: Ka represents Karup, S represents spring application, and C represents pesticide C. MAC is the maximum allowed concentration 0.1μg/l.
4.3. Summary and Concluding Remarks
The choice of application date and plant evolution were found to affect some of the estimated leaching output, and may thus influences which type of pesticides will pass the Danish pesticide approval system.
While pesticide A and C were only minor affected by application date, a major impact was observed for pesticide B when applied on the Tylstrup scenario. By applying the pesticides ~three weeks later in the spring/earlier in the fall the leachate concentration 1 m b.g.s. of pesticide B increases with up to a factor 100 from below to beyond the maximum allow concentration 0.1 mg/l. A new leaching pattern can thus appear by choosing another application date and plant evolution.
Generally, the water and mass balance at the PLAP scenarios were not very sensitive to the change in application date and plant evolution. It should though be noticed that a drastic increase in drainage at the Estrup scenario with spring cereals was observed.
5. New Model Scenarios for the Registration Procedure of Pesticides
- 5.1. Selection of New Model Scenarios
- 5.2. Description of Themes included in the Standard of Reference
5.1. Selection of New Model Scenarios
As background for the selection of a new model scenario for pesticide approval respectively for a sandy and clayey soil among the 4 sandy sites (Karup, Hamburg, Tylstrup, and Jyndevad) and clayey sites (Langvad, Silstrup, Estrup, and Faardrup), a standard of reference is establish, Table 8. The standard of reference includes a characterisation of all the scenarios with regard to observation in relation to precipitation, geology, and reliability of field data and conceptual model (Theme A-F in Table 8) and the leaching results of the MACRO-simulations presented in this report (Theme G-I in Table 8). In this connection it should be emphasised that the geological themes B-D’s influence of pesticide leaching are unknown. The standard of references is summarised in Table 8, whereas a detailed description of the individual themes, and why they are chosen as themes, is given in section 5.2.
Based on this standard of reference, the Jyndevad and the Silstrup are recommended as the new model scenarios for sand and clay, respectively. This recommendation is based on the following evaluation of the standard of reference:
The Jyndevad scenario represents the sand-scenario with the highest observed average precipitation and highest degree of simulated A, B, and C-leaching. Additionally Jyndevad represents a ‘Moorland Plain’ with topsoil similar to about 24% of the topsoils covering Denmark. The surface geology of Jyndevad is characterised by ‘Glaciofluvial sand and gravel’, which only represents 2% of the surface geology of Denmark. By choosing the Karup scenario instead of the Jyndevad scenario, the surface geology of Denmark will be represented by 10%, however the reliability of the field data and conceptual model behind this scenario is low. The same lack of reliability is present for the Hamburg scenario, added with no information concerning the geological environment beside sparse texture information. The Tylstrup scenario has as reliable field data and conceptual model as the Jyndevad scenario, though it represents Yoldia Beds, which only covers 2% of the land surface of Denmark. According to Greve et al. (2007), the spatial variation in texture on a ‘Yoldia Bed’ is much higher than for other element. Given this high spatial variation, an increased uncertainty in the simulated leaching of the Tylstrup scenario could be introduced given that it is based on a one dimensional conceptual model.
The Silstrup scenario represents the clay-scenario with the second highest observed average precipitation and a high degree of simulated A, B, and C-leaching. Additionally, Silstrup represents a ‘Terminal Moraine Landscape’ with topsoil, which has the same soil type as only 5% of the topsoils covering Denmark. The surface geology of Jyndevad is like the other clay-scenarios characterised by ‘Till clayey and fine sandy’, which represents 38% of the surface geology of Denmark. If choosing the Langvad scenario instead of the Silstrup scenario, the soil type of the topsoil of Denmark will be represented by 28%, however the reliability of the field data and conceptual model behind this scenario is low. The Faardrup scenario has as reliable field data and conceptual model as the Silstrup scenario, though it represents the lowest observed average precipitation, the lowest degree of simulated A, B, and C-leaching, and hereby not a worst case scenario. Contrary, the Estrup has the highest observed average precipitation and a high degree of simulated A, B, and C-leaching. However being a ‘Hill Island’, which according to Greve et al. (2007) is characterised by large spatial variation in texture, an increased uncertainty in the simulated leaching of the Estrup scenario could be introduced given that it is based one dimensional conceptual model. Given errors in the TDR-measurements, the reliability of the field calibration data and conceptual model of Estrup is slightly lower than at e.g. Silstrup.
| Sand scenarios | Clay scenarios | |||||||
| Theme | Karup | Hamburg | Tylstrup | Jyndevad | Langvad | Silstrup | Estrup | Faardrup |
| BASED ON OBSERVATIONS | ||||||||
| A. Average precipitation (mm) | 912 | 786 | 850 | 1024 | 675 | 915 | 1032 | 653 |
| B. Surface geology and its represen- tativity (%). |
Down- wash sandy deposits 10% |
? | Glacio- marine clay, silt, and sand 19% |
Glacio- fluvial sand and gravel 2% |
Till clayey and fine- sandy 38% |
Till clayey and fine- sandy 38% |
Till clayey and finesandy 38% |
Till clayey and fine-*sandy 38% |
| C. Soil type of topsoil and its represen- tativity (%) |
JB3 - 9% | JB4 - 19% | JB2 - 8% | JB1 - 24% | JB5/6 - 28% | JB7 – 5% | JB5/6 - 28% | JB5/6 - 28% |
| D. Element of the landscape and its represen- tativity (%). |
Moorland Plain 11% |
? | Yoldia Beds 2% |
Moorland Plain 11% |
Younger Moraine Land- scape ~30% |
Terminal Moraine Land- scape ~30% |
Hill Islands 11% |
Younger Moraine Land- scape ~30% |
| E. Reliability of field data (Quality; Quantity). | (+; +) | (+; +) | (+++; ++) | (+++; ++) | (+; +) | (+++; +++) | (+++; ++) | (+++; +++) |
| F. Reliability of conceptual model (Water Balance; Mass Balance of bromide). |
(+; - ) | (+; - ) | (++; ++) | (++; ++) | (+; - ) | (+++; +++) | (++; ++) | (+++; +++) |
| BASED ON SIMULATIONS | ||||||||
| G. Degree of simulated pesticide A leaching. Spring application (1 m b.g.s.; 3 m b.g.s.; Drain). Fall application (1 m b.g.s.; 3 m b.g.s.; Drain). Number of annual pesticide concen- trations 1 m b.g.s. ≥ 0.1 μg/l - Spring/Fall. 3 m b.g.s. ≥ 0.1 μg/l - Spring/Fall. |
(+++; - ; - ) (+++; - ; - ) 20/20 ? |
(+++; - ; - ) (+++; - ; - ) 20/20 ? |
(+++; +++ ; -) (+++; +++; - ) 20/20 20/20 |
(+++; +++ ; -) (+++; +++; - ) 20/20 20/20 |
(+++; - ; + ) (+++; - ; + ) 20/20 ? |
(+++;+ ++;++) (+++; +++; +++) 20/20 20/20 |
(+++;+ ++;++) (+++; +++; +++) 20/20 20/20 |
(+++; +++;+) (+++; +++;+) 20/20 17/20 |
| H. Degree of simulated pesticide B leaching. Spring application (1 m b.g.s.; 3 m b.g.s.; Drain). Fall application (1 m b.g.s.; 3 m b.g.s.; Drain). Number of annual pesticide concen- trations 1 m b.g.s. ≥ 0.1 μg/l - Spring/Fall. 3 m b.g.s. ≥ 0.1 μg/l - Spring/Fall. |
(+; - ; - ) (+; - ; - ) 0/20 ? |
(+; - ; - ) (+; - ; - ) 0/6 ? |
(+; + ; - ) (++; +; - ) 0/6 0/0 |
(++; ++ ; - ) (+++; +++; - ) 1/20 1/20 |
(++; - ; + ) (+++; - ; + ) 6/20 ? |
(++; ++ ; + ) (+++;+ ++;++) 14/20 13/20 |
(++;+ +;+) (+++; +++; +++) 14/20 8/20 |
(+;+;+) (++; ++;+) 0/8 0/5 |
| I. Degree of simulated pesticide C leaching. Spring application (1 m b.g.s.; 3 m b.g.s.; Drain). Fall application (1 m b.g.s.; 3 m b.g.s.; Drain). Number of annual pesticide concen- trations 1 m b.g.s. ≥ 0.1 μg/l - Spring/Fall. 3 m b.g.s. ≥ 0.1 μg/l - Spring/Fall. |
(+; - ; - ) (+; - ; - ) 0/20 ? |
(+; - ; - ) (+; - ; - ) 0/20 ? |
(+; + ; - ) (+; +; - ) 0/0 0/0 |
(+++; +++;-) (+++; +++;-) 20/20 18/20 |
(++;- ;+) (+++;- ;+) 20/20 ? |
(+++;++ +;++) (+++;++ +;++) 20/20 20/20 |
(+++;+ ++;++) (+++;+ +;++) 20/20 15/10 |
(+;+;+) (+;+;+) 0/0 0/0 |
Table 7. Standard of reference - a characterisation of all the scenarios with respect to precipitation, geology, reliability of field data/conceptual model, and simulated pesticide leaching
5.2. Description of Themes included in the Standard of Reference
The themes A-I included in the standard of reference, Table 8, are chosen within two categories: ‘Based on observations’ and ‘Based on simulations’. The category ‘Based on observations’ is included to emphasis the need of getting well-characterised scenarios as model scenario for pesticide approval. To evaluate the outcome of the MACRO-simulations it is important to be aware of the climatic and geological environment of the chosen scenarios.
The first Theme A is the average precipitation of the scenarios. The geographical variation in precipitation across Denmark, Figure 12., needs to be taken into account when selecting scenarios for pesticide approval. Areas with the highest mean annual precipitation and hereby a higher leaching risk are located in the Western part of Denmark, wherefrom there is a reduction in the mean annual precipitation of up to several hundreds millimetre going East. Langvad and Faardrup therefore represent the lowest average precipitation. The yearly precipitation of the 8 scenarios in question are within the range of approximately 400-1400 mm, Figure 12., and the simulated yearly net precipitation is within the range of approximately 20-900 mm, Figure 4.
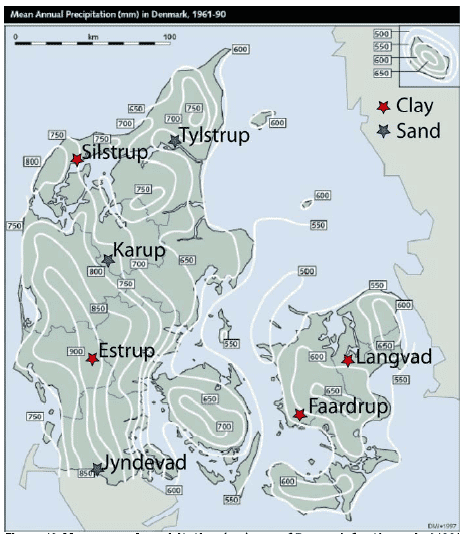
Figure 12. Mean annual precipitation (mm) map of Denmark for the period 1961-1990 (Frich et al., 1997) with the location of the Danish sand scenarios (Karup, Tylstrup and Jyndevad) and clay scenarios (Langvad, Silstrup, Estrup, and Faardrup).
Theme B is addressing the surface geology of the scenarios and its representativity. A representative surface geology map for respectively the Danish sand and clay scenarios are given in Figure 13. The map provides large scale information concerning genetic facies 1 m b.g.s., which renders expectations to soil property above 1 metre and hereby interpretation to hydraulic conditions and presence of chalk/humus/fractures/biopores.
The Danish scenarios broadly represent the Danish surface geology. The three sand scenarios Karup, Tylstrup, and Jyndevad represent three different surface geology in the given order: ‘Downwash sandy deposits’, ‘Glaciomarine clay, silt and sand’, and ‘Glaciofluvial sand and gravel‘, which cover respectively approximately 10%, 2%, and 19% of Denmark’s land area – A variation, which needs to be taken into account in the selection of the new sand-model-scenarios for pesticide approval. The four clay scenarios Langvad, Silstrup, Estrup and Faardrup all represent the same surface geology ‘Till, clayey and fine-sandy’, which covers ~38% of Denmark’s land.
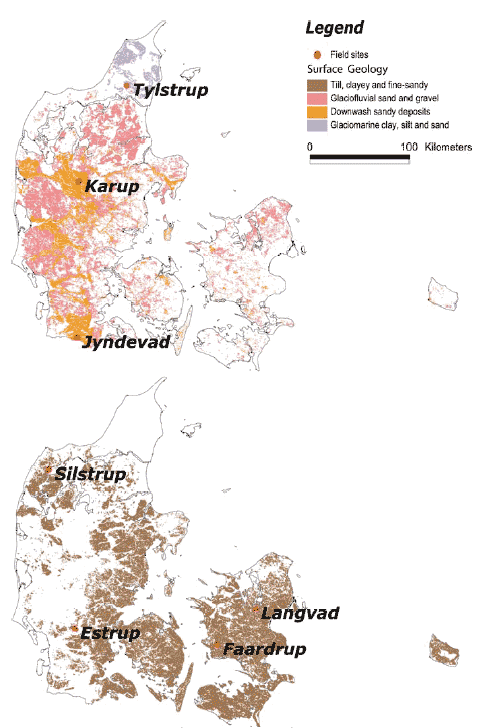
Figure 13. Representative surface geology maps for respectively the Danish sand scenarios (Karup, Tylstrup and Jyndevad) and clay scenarios (Langvad, Silstrup, Estrup, and Faardrup) included in this work. These maps are based on the Geological Survey of Denmark and Greenland’ digital map of the surface geology of Denmark, 1:25.000 (see www.geus.dk).
Theme C concerns the soil type of the topsoil of the scenarios and its representativity, Figure 14. The texture of the topsoil is very important for the amount of pesticides leaching through the soil, since the protection offered by the subsoil layers are considered to be low for most pesticides, due to the very low microbial activity, sorption and degradation generally found in these layers (Aamand et al., 2004). Iversen et al. (2006) have described the leaching risk of pesticides in sandy-soil in relation to its texture, which showed an increase in MACRO simulated leaching risk with a decreasing humus, and clay+silt content. For that reason it is important to incorporate the knowledge concerning the soil texture of the top soil in the selection of new model-scenarios.
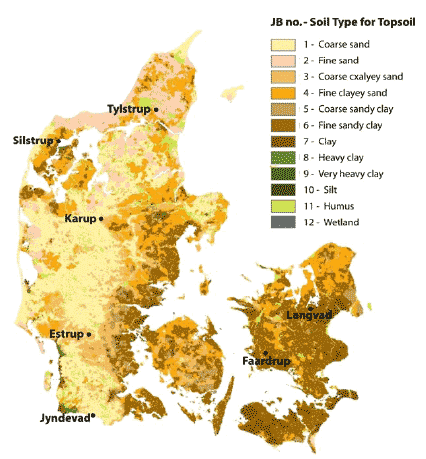
Figure 14. The Danish Institute of Agricultural Sciences’ nationwide topsoil property map of Denmark. The map is based on the texture classes (JB-numbers) of the Danish Soil Classification (Greve et al., 2005).
Theme D is the element of landscape of the scenarios and its representativity (Madsen et al., 1992). The map of the elements of the landscape illustrates the distribution of genetic facies at large scale and hereby renders expectations to soil properties in areas not covered by other geological data. This includes e.g. interpretation on hydraulic properties and presence of humus/fractures/biopores.
Figure 15 shows the different element of the Danish landscape and the element of landscape that each of the eight scenarios represent is given in Table 8. Results from the KUPA-project show that the element of landscape like ‘Yoldia Beds’ (Tylstrup scenario) and ‘Hill Island’ (Estrup scenario) give rise to a large variation within the texture (Greve et al., 2007). Given this variation, the representativeness of a conceptual 1-D model based on 3-D field observations gives rise to a large uncertainty.
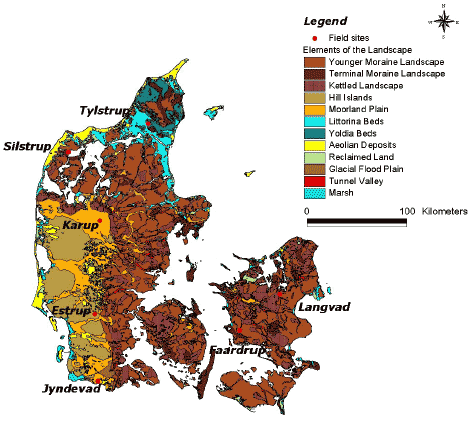
Figure 15. The Danish Institute of Agricultural Sciences’ map on Element of the landscape added locations of Danish sites representing the scenarios: Karup, Tylstrup, Jyndevad, Langvad, Silstrup, Estrup, and Faardrup.
Theme E addresses the reliability of field data in relation to quality and quantity, which is essential for setting up realistic conceptual models for simulating pesticide leaching. The reliability is categorised into three groups describing both quality and quantity of available field data. Quantity “+”, “++” and “+++” refers to minimal, medium and large data availability, respectively. Likewise for quality where “+”, “++” and “+++” refers to low, medium and high data quality, respectively. In the PLAP-project, field data of high quality is collected during a 5 years period, Appendix C. At the two sand scenarios of PLAP (Tylstrup and Jyndevad), less information is available concerning the overall water and the mass balance, given that no data on water fluxes are present and only point measurement are obtained. For the three clay scenarios of PLAP (Silstrup, Estrup, and Faardrup), detailed drain information provides venerable information on water fluxes, why a calibration against these field data provides a more reliable conceptual model. In Estrup, some TDR-data errors are present resulting in a less reliable conceptual model than for Silstrup and Faardrup. Compared to the PLAP scenarios, the field data behind Hamburg, Karup and Langvad seems to be minimal both in quality and quantity.
Theme F is linked directly to theme E and addresses the reliability of the conceptual models. Given the varying quality and quantity of field data for the eight scenarios, the reliability of conceptual models for the scenarios is varying a lot. For the PLAP-scenarios, the calibration results are shown in Appendix C and summarised in Table C1, which illustrate how well the final conceptual model is performing compared to the direct field measurements. The conceptual models behind the Hamburg, Karup and Langvad scenarios are not calibrated against any direct field measurements, why the reliability of these models is minimal.
Theme G-I addresses the degree of simulated leaching of the eight scenarios applying the three pesticides (A, B and C). Based on the results presented in section 3.3.2 - 3.4 the average yearly leaching is ranked in following three categories:
+ Pesticide concentration < 0.01 μg/l,
++ Pesticide concentration < 0.1 μg/l and >0.01 μg/l,
+++ Pesticide concentration ≥ 0.1 μg/l,
Results are presented separately for spring and fall application in respectively 1 m b.g.s., 3 m b.g.s., and drains. Additionally, the number of annual pesticide concentrations at 1 and 3 m b.g.s. equal or larger than maximum allowed concentration for respectively the spring and the fall application is given in the format of 18/20 (spring/fall).
6. Conclusions
This study evaluates the reliability and representativeness of both the existing model scenarios (Langvad, Karup, and Hamburg) and the recent PLAP scenarios (Jyndevad, Tylstrup, Silstrup, Estrup, and Faardrup) with the purpose of recommending the two scenarios to be used in the coming registration procedure.
The PLAP scenarios Jyndevad (sand) and Silstrup (clay) are proposed for future use in the Danish pesticide approval procedure. Given the lack of criteria defined by the Danish EPA, this recommendation is based on characterization with respect to precipitation, geology, reliability of field data and conceptual model. Additionally it is based on the authors´ assumption that choosing the worst case scenarios with respect to leaching will best protect the Danish groundwater against pesticides.
The existing scenarios (Karup, Langvad, and Hamburg) were found to be less restrictive than some of the PLAP scenarios. For the sandy scenarios, the highest leaching was found at Jyndevad. The somewhat lower leaching at Karup resembles that of Tylstrup, while that of Hamburg is generally lower. For clay scenarios the leaching at Langvad is higher than that at Faardrup but considerably lower than that of Silstrup and Estrup. These conclusion were based upon modelling results covering a 20 years period where 1 kg/ha of pesticides was applied every year to either spring cereals or winter cereals. Crop parameters, dose and application time of pesticides and pesticide properties were kept the same in all scenarios. The numerical model used for setting up the scenarios comprised PELMO 3.2 (Hamburg), MACRO 4.4.2 (Langvad and Karup) and the recent MACRO 5.1 (the PLAP-scenarios: Tylstrup, Jyndevad, Silstrup, Estrup, and Faardrup).
Often, when comparing pesticide leaching for different scenarios, the question arises, if the ranking of scenarios with respect to leaching is dependant on e.g. choice of pesticide and application date linked with plant evolution. The latter is studied in a sensitivity analysis for the five PLAP scenarios, where the application dates are changed approximately three weeks. Changing the application date and related plant evolution may influence pesticide leaching and subsequent the approval of pesticides.
7. Perspectives
When assessing the reliability of the selected scenarios it should be noted that they have not been validated against measured pesticide leaching data. While calibration made against measured groundwater table, soil water contents, bromide concentration, and for the clay soils drainage runoff suggested a good model description of water and conservative solute transport, the predicting capability towards pesticide leaching has not yet been evaluated for the PLAP scenarios. Since the start of this project measured pesticide data has been made available at the PLAP sites. Since now possibly, a validation testing the capability of the model to predict pesticide leaching, is highly recommended to attach further reliability to model output.
When assessing the representativeness of the selected scenarios is should be noted that a present correlation between leaching and e.g. precipitation, surface geologies, soil types, and elements of landscapes has not yet been recognised. Establishing these types of correlation would be valuable for attaching further confidence in assessing the representativeness of the selected scenarios.
References
Abbaspour, K. C., van Genuchten, M. T., Schulin, R., and Schäppi, E., 1997. A sequential uncertainty domain inverse procedure for estimating subsurface flow and transport parameters, Water Resources Research 33(8):1879-1892.
Allerup, P., and Madsen, H., 1979. Accuracy of point precipitation measurements. Danish Meteorological Institute, Climatological Papers No. 5, Copenhagen, 84 pp.
Aamand, J, Rasmussen, J.J., and Jacobsen, O. S. 2004: Opdeling af pesticider i forhold til deres sorptions- and nedbrydningsegenskaber. Koncept for Udpegning af Pesticidfølsomme Arealer, KUPA (In Danish), Geological survey of Denmark and Greenland 2004, 33 pp.
Aslyng, H. C., and Hansen, S., 1982. Water balance and crop production simulation. Hydrotechnical Laboratory. The Royal Veterinary and Agricultural University, Copenhagen, 200 pp.
Barlebo, H. C., Hansen, M., Greve, M., Waagepetersen, R., and Lindhardt, B., 1999. Udvaskning af pesticider: Modellering af betydningen af variation i danske jordarter, nedbør og stofparametre. GEUS Rapport 1999/40, 101 pp.
Boesten, J.J.T.I., 1991. Sensitivity analysis of a mathematical model for pesticide leaching to groundwater. Pesticide science 31(3):375-388.
Dubus, I.G., and Brown, C.D., 2002. Sensitivity and First-Step Uncertainty Analyses for the Preferential Flow Model MACRO. Journal of Environmental Quality 31: 227-240.
European Commission, E1 - Plant health, 2003. Guidance document on the assessment of the relevance of metabolites in groundwater of substances regulated under council directive 91/414/EEC (Sanco/221/2000 –rev.10, 25 February 2003).
EU Working documents from the European Commision, EU Review Program.
FOCUS, 1995. Boesten, J. et al: Leaching Models and EU Registration. The final report of the work of the Regulatory Modelling Work Group of FOCUS. European Commission Document 4952/VI/95.
FOCUS, 2000. FOCUS groundwater scenarios in the EU review of active substances. Report of the FOCUS Groundwater Scenarios Workgroup, EC Document Reference SANCO/321/200 rev.2, 202 pp.
FOCUS, 2002. Generic guidance for FOCUS groundwater scenarios. Version 1.1. April 2002.
Frick, P., Rosenørn, S., Madsen, H., and Jensen, J.J., 1997. Observed Precipitation in Denmark, 1961-90. Danish Metrological Institut. Technical report 97-8. ISSN 0906-897.
GEUS, 2004. Særligt pesticidfølsomme sandområder: Forudsætninger og metoder for zonering. Koncept for udpegning af pesticidfølsomme Arealer. Danmarks og Grønlands Geologiske Undersøgelse (In Danish).
Greve, M.H., Greve, M.B., Bøcker, P.K., Balstrøm, T., and Madsen, H.B., 2006. Generation of a raster based nationwide topsoil property map of Denmark combining choropleth maps and point information. Conference contribution to GeoEny 2006, 6th International conference on Geostatistics for Environmental Applications, Rhodos, Greece.
Iqbal, M., 1983. An introduction to solar radiation. Academic Press.
Iversen, B.V., Greve, M.B., and Nygaard, E., 2006. Sandede områders pesticidfølsomhed (In Danish). Vand & Jord 13(4).
Greve, M. et al., 2007. Mapping intrinsic vulnerability to leaching of pesticides in sandy areas. (In prep.)
Jørgensen, P.R., Klint, K.E.S., and Kistrup, J.P., 2003. Monitoring Well Interception with Fractures in Clayey Till. Ground Water 41(6): 772-779.
Kjær, J., Ullum, M., Olsen, P., Sjelborg, P., Helweg, A., Mogensen, B., Plauborg, F., Grant, R., Fomsgaard, I., and Brüsch, W., 2003. The Danish Pesticide Leaching Assessment Programme: Monitoring results, May 1999–July 2002, Geological Survey of Denmark and Greenland.
Kjær, J., Olsen, P., Barlebo, H. C., Henriksen, T., Juhler, R. K., Plauborg, F., Grant, R., Nygaard, P., and Gudmundsson, L., 2005. The Danish Pesticide Leaching Assessment Programme: Monitoring results, May 1999–July 2004, Geological Survey of Denmark and Greenland.
Kjær, J., Olsen, P., Rosenbom, A.E., Barlebo, H. C., Henriksen, T., Juhler, R. K., Plauborg, F., Grant, R., Nygaard, P., and Gudmundsson, L., 2007. The Danish Pesticide Leaching Assessment Programme: Monitoring results, May 1999–July 2006, Geological Survey of Denmark and Greenland (In prep).
Kördel, W., Klöpppel, H., and Hund, K., 1989. Physikalisch-chemische und biologische Charakterisierung von Böden zur Nutzung in Versickerungsmodellen von Pflanzeenschutsmitteln. (Abschlussbericht. Fraunhofer-Institut für Umweltchmie und Ökotoxikologie, D-5948 Schmallenberg-Grafschaft, 1989). (In Germann)
Larsbo, M., and Jarvis, N., 2003. Macro 5.0: A model of water flow and solute transport in macroporous soil. Technical description. Swedish University of Agricultural Sciences, Department of Soil Sciences, Uppsala, pp. 52, http://www.mv.slu.se/bgf/.
Linders, J.B.H.J, Jansma, J.W., Mensink, B.J.W.G. and Otermann, K. (1994). Pesticides: Benefaction or Pandora´s box. National Institute of Public Health and Environmental Protection, The Netherlands. Report no. 679101014.
Lindhardt, B., Abildtrup, C., Vosgerau, H., Olsen, P., Torp, S., Iversen, B.V., Jørgensen J. O., Plauborg, F., Rasmussen, P., and Gravesen, P., 2001. The Danish Pesticide Leaching Assessment Programme: Site characterization and monitoring design, Geological Survey of Denmark and Greenland.
Madsen, H.B, Nørr, A.H., Holst, K.A., 1992. Atlas of Denmark, Seri I, Bind 3, Den Danske jordklassificering. Det Kongelige Danske Geografiske Selskab. Rosendahls Bogtrykkeri. C.A. Reitzel.
Miljøstyrelsen, 1997. Guidance Paper for Using Mathematical Models in an Assessment of Pesticide Mobility. Released November 28, 1997.
Miljøstyrelsen, 1998. Vejledning for anvendelse af matematiske modeller til bedømmelse af pesticiders mobilitet. (In Danish)
Miljøstyrelsen, 1999. Evaluation of mathematical modelling of risk of ground water pollution. Released May 28, 1999.
Petersen, C.T., Holm, J., Koch, C.B., Jensen, H., and Hansen, S., 2002. Movement of pendimethalin ioxynil and soil particles to field drainage tiles. Pest Management Science 59: 85-95.
Roberts, T. (1998). Metabolic Pathways of Agrochemicals. Part one – Herbicides and Plants Growth Regulators. The Royal Society of Chemistry, Cambridge, UK. (ISBN 0-85404-494-9)
Storm, B., Styczen, M., and Clausen, T., 1990. Regional Model for Nitrate Transport and Transformation. NPo Research in the NAEP, Report B15. National Agency of Environmental Protection, Copenhagen (In Danish).
Styczen, M., Petersen, S., Kristensen, M., Jessen, O. Z., Rasmussen, D., Andersen, M. B., and Sørensen, P. B., 2004. Calibration of Models Describing Pesticide Fate and Transport in Lillebæk and Odder Bæk Catchment. Pesticide Research, No. 62. Danish Environmental Protection Agency.
Tomlin, C.D.S. (1997). The Pesticide Manual – Eleventh Edition. British Crop Protection Council, Berks, UK. (ISBN 1 901396 11 8)
Appendix A Description of Karup and Langvad Scenarios
1.1 Conceptual Model
In both scenarios, the conceptual model includes a 2.5 meter soil column separated into four horizons. The Karup site consists of sandy soil, which is considered rather homogeneous neglecting macropore flow. It is situated by the stream Karup Å, why the lower boundary is defined as a constant potential close to field capacity. This approach mimics the free drainage conditions in a deep unsaturated profile overlying a sandy aquifer. The Langvad site consists of loamy soil with macropores, why macropore flow is considered with the macroporosity decreasing from 2% in the top soil to 1% in the deepest horizon. Since the site is situated by the stream Langvad Å, the lower boundary is defined as having a constant gradient, which was calibrated in order to ensure realistic fluctuations of the groundwater table. This approach mimics the situation with shallow topsoil overlaying an aquifer in 2.5 m depth. Artificial drains are installed 1.3 m b.g.s. (Miljøstyrelsen, 1998).
1.2 Data
1.2.1 Climate
Daily climatic data comprising precipitation, min. and max. temperatures and sunshine hours for the two locations were used (ref. Danish Meteorological Institute). The precipitation data were corrected for wind and wetting losses according to Allerup and Madsen (1979), and it was ensured that the annual rainfall compiled with the area mean values, which were originally used for the water balance calculations in Storm et al. (1990). Potential evaporation was calculated using a modified Makkink approach (Aslyng and Hansen, 1982) with the use of radiation data calculated from sunshine hours using the Ångström formula (Iqbal, 1983). Min. and max. air temperatures were used directly. In Table A1 annual precipitation and potential evaporation for the two locations are shown (Miljøstyrelsen, 1998).
Table A1. Climatic data available for Karup and Langvad.
| Year | Karup | Langvad | ||
| Precipitation (mm/year) |
Potential Evaporation (mm/year) |
Precipitation (mm/year) |
Potential Evaporation (mm/year) |
|
| 1968 | 895 | 611 | - | - |
| 1969 | 647 | 593 | - | - |
| 1970 | 848 | 609 | - | - |
| 1971 | 702 | 625 | - | - |
| 1972 | 714 | 609 | - | - |
| 1973 | 650 | 625 | - | - |
| 1974 | 862 | 656 | 795 | 645 |
| 1975 | 694 | 649 | 622 | 677 |
| 1976 | 640 | 632 | 609 | 666 |
| 1977 | 941 | 613 | 606 | 635 |
| 1978 | 811 | 574 | 857 | 612 |
| 1979 | 939 | 566 | 662 | 592 |
| 1980 | 1064 | 577 | 805 | 604 |
| 1981 | 1078 | 583 | 769 | 618 |
| 1982 | 891 | 614 | 598 | 667 |
| 1983 | 1017 | 612 | 626 | 630 |
| 1984 | 855 | 607 | 680 | 619 |
| 1985 | 927 | 600 | 689 | 604 |
| 1986 | 904 | 598 | 620 | 611 |
| 1987 | 1044 | 595 | 692 | 575 |
| 1988 | 992 | 607 | 639 | 623 |
| 1989 | 828 | 645 | 562 | 648 |
| 1990 | 1053 | 627 | 650 | 661 |
| 1991 | 901 | 642 | 697 | 628 |
| 1992 | 941 | 685 | 509 | 669 |
| 1993 | 855 | 615 | 818 | 626 |
| 1994 | 1062 | 627 | 744 | 662 |
| 1995 | 799 | 634 | 533 | 660 |
| 1996 | 590 | 614 | 424 | 644 |
1.2.2 Soil
The soil properties and parameters for the Karup and the Langvad groundwater scenario used as input parameters for MACRO 4.4.2. are presented in Table A2- A5.
Table A2. MACRO soil properties for the Karup groundwater scenario
| Property | Ap horizon | B1 horizon | B2 horizon | BC horizon |
| Thickness [cm] | 20 | 20 | 30 | 180 |
| Texture | Loamy sand | Sand | Sand | Sand |
| Bulk density [g/cm³] | 1.51 | 1.55 | 1.55 | 1.53 |
| Organic carbon [%] | 2.2 | 1.7 | 0.3 | 0.2 |
| PH | -99 | -99 | -99 | -99 |
| Structure | Weak fine granular | Structureless | Structureless | structureless |
Table A3. MACRO soil parameters for the Karup groundwater scenario.
| Parameters | Ap horizon | B1 horizon | B2 horizon | BC horizon |
| Thickness [cm] | 20 | 20 | 30 | 180 |
| Saturated conductivity [mm h-1] | 200.00 | 125.00 | 125.00 | 720.00 |
| Boundary conductivity [mm h-1] | 1.000 | 2.000 | 2.000 | 10.000 |
| Boundary tension [cm] | 15 | 15 | 15 | 10 |
| Bulk density [g cm-3] | 1.51 | 1.55 | 1.55 | 1.53 |
| Saturated water content [m³ m-3] | 0.420 | 0.420 | 0.420 | 0.420 |
| Boundary water content [m³ m-3] | 0.340 | 0.320 | 0.320 | 0.320 |
| Wilting point [m³ m-3] |
0.100 | 0.060 | 0.060 | 0.040 |
| Effective aggregate half-width [mm] | 1 | 1 | 1 | 1 |
| Pore-size distribution index (mic.) | 0.200 | 0.290 | 0.290 | 0.720 |
| Pore size distribution index (mac.) | 6.0 | 6.0 | 6.0 | 6.0 |
Table A4. MACRO soil properties for the Langvad groundwater scenario.
| Property | Ap horizon | Eb horizon | Ebg horizon | BCg horizon |
| Thickness [cm] | 20 | 20 | 30 | 180 |
| Texture | Sandy loam | Sandy loam | Sandy loam | Sandy loam |
| Bulk density [g/cm³] | 1.5 | 1.65 | 1.7 | 1.76 |
| Organic carbon [%] | 2.1 | 1.4 | 0.5 | 0.3 |
| pH | -99 | -99 | -99 | -99 |
| Structure | Moderate medium blocky | Weak medium blocky | Weak medium blocky | Weak coarse prismatic |
Table A5. MACRO soil parameters for the Langvad groundwater scenario.
| Parameters | Ap horizon | Eb horizon | Ebg horizon | BCg horizon |
| Thickness [cm] | 20 | 20 | 30 | 180 |
| Saturated conductivity [mm h-1] | 90.00 | 360.00 | 360.00 | 26.00 |
| Boundary conductivity [mm h-1] | 0.500 | 0.100 | 0.100 | 0.080 |
| Boundary tension [cm] |
10 | 12 | 12 | 25 |
| Bulk density [g cm-3] | 1.50 | 1.65 | 1.70 | 1.76 |
| Saturated water content [m³ m-3] |
0.450 | 0.360 | 0.360 | 0.340 |
| Boundary water content [m³ m-3] |
0.430 | 0.340 | 0.340 | 0.330 |
| Wilting point [m³ m-3] |
0.140 | 0.120 | 0.120 | 0.150 |
| Effective aggregate half-width [mm] | 20 | 20 | 20 | 20 |
| Pore-size distribution index (mic.) | 0.150 | 0.150 | 0.150 | 0.150 |
| Pore size distribution index (mac.) | 5.0 | 5.0 | 5.0 | 5.0 |
Appendix B Description of Hamburg Scenario
1.1 Conceptual Model
The conceptual model given in the FOCUS groundwater scenario for Hamburg describes a 2 m deep sandy soil column containing six different horizons. This sandy media is rather homogeneous and macropore flow is not considered.
1.2 Data
The climate and soil data for the Hamburg scenario are described in "FOCUS groundwater scenarios in the EU review of active substances" - The report of the work of the Groundwater Scenarios Workgroup of FOCUS (FOCUS, 2000). (http://viso.ei.jrc.it/focus/gw/).
1.2.1 Climate
The precipitation and the mean annual temperature are within the range of 601 to 800 mm and 5 to 12.5°C, respectively. More information can be found in Kördel et al. (1989).
Figure 1 and 2 illustrate PELMO´s FOCUS-standard output for the annual water balance in mm separated into evapotranspiration (volatilisation), runoff (surface runoff) and percolation for use of respectively winter and spring cereals. The figures show that for the application of the winter cereals instead of spring cereals will give a larger percolation through the soil, less surface runoff, and less evaporation. The origin of these climate data is not clear and is not to be found in Kördel et al. (1989).
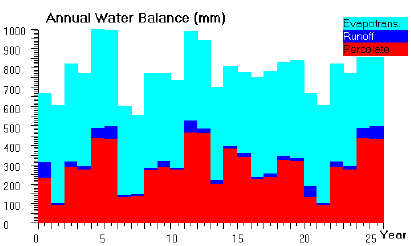
Figure 1. Annual water balance including evapotranspiration, runoff and percolation for the Hamburg scenario when applying winter cereals annually.
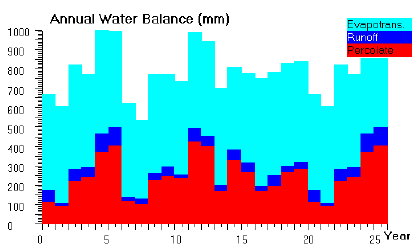
Figure 2. Annual water balance including evapotranspiration, runoff and percolation for the Hamburg scenario when applying spring cereals annually.
1.2.2 Soil
The soil texture and hydraulic properties incorporated in PELMO for the six horizons are described in Table B1 and B2. The Hamburg soil profile has a very low organic matter content below a depth of 60 cm, which could increase the leaching to the groundwater (Boesten, 1991).
Table B1. Soil texture (FOCUS groundwater scenarios in the EU review of active substances)
| Horizon | Depth | Classification | pH- H2O |
pH- KCl |
Texture | om | oc | Bulk density | Depth Factor |
||
| Cm | <2 mm [%] |
2-50 mm [%] |
>50 mm [%] |
[%] | [%] | [gcm-3] | [-] | ||||
| Ap | 0-30 | Sandy loam | 6.4 | 5.7 | 7.2 | 24.5 | 68.3 | 2.6 | 1.5 | 1.5 | 1.0 |
| BvI | 30-60 | Sandy loam | 5.6 | 4.9 | 6.7 | 26.3 | 67 | 1.7 | 1 | 1.6 | 0.5 |
| BvII | 60-75 | Sand | 5.6 | 4.9 | 0.9 | 2.9 | 96.2 | 0.34 | 0.2 | 1.56 | 0.3 |
| Bv/Cv | 75-90 | Sand | 5.7 | 5.0 | 0.0 | 0.2 | 99.8 | 0.0 | 0.0 | 1.62 | 0.3 |
| Cv | 90-100 | Sand | 5.5 | 4.8 | 0.0 | 0.0 | 100 | 0.0 | 0.0 | 1.6 | 0.3 |
| Cv | 100-200 | Sand | 5.5 | 4.8 | 0.0 | 0.0 | 100 | 0.0 | 0.0 | 1.6 | 0.0 |
Table B2. Soil hydraulic properties, Van Genuchten/Mualem parameters. *AW: Plant available water in the layer.
| Depth | qs | qs | a | N | M | Water content | Ksat | l | AW* | |
| 10kPa | 1600kPa | |||||||||
| [m³m-3] | [m³m-3] | [m-1] | - | - | [m³m-3] | [m³m-3] | [ms-1] | - | [mm] | |
| 0-30 | 0.3910 | 0.0360 | 1.491 | 1.4680 | 0.3188 | 0.292 | 0.064 | 23.330 | 0.500 | 68.4 |
| 30-60 | 0.3700 | 0.0300 | 1.255 | 1.5650 | 0.3610 | 0.277 | 0.047 | 31.670 | 0.500 | 69.0 |
| 60-75 | 0.3510 | 0.0290 | 1.808 | 1.5980 | 0.3742 | 0.229 | 0.040 | 28.330 | 0.500 | 28.4 |
| 75-90 | 0.3100 | 0.0150 | 2.812 | 1.6060 | 0.3773 | 0.163 | 0.022 | 28.330 | 0.500 | 21.2 |
| 90-100 | 0.3100 | 0.0150 | 2.812 | 1.6060 | 0.3773 | 0.163 | 0.022 | 28.330 | 0.500 | 14.1 |
| 100-200 | 0.3100 | 0.0150 | 2.812 | 1.6060 | 0.3773 | 0.163 | 0.022 | 28.330 | 0.500 | |
Appendix C Calibration of PLAP Models
1.1 Calibration of PLAP Scenarios
The 1-dimensional MACRO model vs. 5.1 (Larsbo and Jarvis, 2003) is applied to the five PLAP sites Tylstrup, Jyndevad, Silstrup, Estrup, and Faardrup covering the soil profile to a depth of 5 m b.g.s., always including the groundwater table. The model is calibrated against water saturation measured in suction cells, depth to groundwater table measured in piezometers, drainage runoff and bromide concentration in water sampled from either suction cups or drainage system during the full monitoring period May 1999 – June 2004. A typical horizontal and vertical lay-out of monitoring devices at a tile-drained site is shown in Figures C1 and C2.
Figure C1. A typical horizontal lay-out of monitoring devices at a tile-drained PLAP site.

Figure C2. A typical vertical lay-out of monitoring devices at a tile-drained PLAP site.
At each site there is spatial heterogeneity. Therefore, the fact that some measurements can be regarded as point measurements and others as integrating over an area has caused the data used in the calibration to be weighted differently. Most emphasis in the calibration has been given to the integrated measurements. They are the groundwater table, measured yearly drainage, and the accumulated bromide leaching in drains.
At the two sand locations there are no integrated measurements of bromide. Instead the bromide transport is calibrated with even emphasis on breakthrough curves at 1 and 2 m b.g.s. from both tracer experiments.
An overview of calibration results is given in Table C1. More detailed information on collected data and the calibration results at each site can be found in the following sections.
Table C1. Overview of calibration results for PLAP scenarios.
| Calibration results | Location | ||||
| Data-type | Tylstrup | Jyndevad | Silstrup | Estrup | Faardrup |
| Flow related: | |||||
| - Depth to groundwater table (GWT) | Level of GWT: Fluctuations: Amplitude less well described |
Level of GWT: Fluctuations: SIM ~ OBS |
Level of GWT: Fluctuations: Initial rise in autumn too late |
Level of GWT: Fluctuations: |
Level of GWT: Fluctuations: |
| - Soil Water Content | |||||
| 0.25 m b.g.s. (SW25) | Level of SW25: SIM often a bit higher than OBS Fluctuations: |
Level of SW25: SIM often a bit higher than OBS Fluctuations: |
Level of SW25: Fluctuations: |
Level of SW25: SIM ~ OBS Fluctuations: Min. OBS not well described |
Level of SW25: SIM ~ OBS Fluctuations: |
| 0.60 m b.g.s. (SW60) | Level of SW60: SIM often a bit higher than OBS Fluctuations: |
Level of SW60: SIM often a bit higher than OBS Fluctuations: |
Level of SW60: Fluctuations: SIM a bit earlier than OBS |
- | Level of SW60: Fluctuations: SIM shape OBS shape |
| 1.10 m b.g.s. (SW110) | Level of SW110: Fluctuations: Min. OBS less well described |
Level of SW110: SIM much lower than OBS Fluctuations: |
Level of SW110: SIM ~ OBS excluding TDR-OBS-errors Fluctuations: Min. OBS less well described excluding TDR-OBS-errors |
- TDR-OBS-errors |
Level of SW110: Fluctuations: |
| - Drainage | - | - | SIM ~ OBS | SIM ~ OBS | SIM ~ OBS |
| Transport related: | |||||
| - Bromide - suction cells | |||||
| 1 m b.g.s. | 1. Peak 2. Peak Breakthrough time: SIM 2 month earlier than OBS Shape/Size: SIM narrower than OBS |
1. Peak 2. Peak Breakthrough time: SIM 4 month too late compared to OBS Shape/Size: SIM narrower than OBS |
Peak Breakthrough time: Shape/Size: SIM amount < OBS amount in June and July 2000 |
Peak Breakthrough time: Shape/Size: SIM ~ OBS |
Peak Breakthrough time: Shape/Size: SIM ~ OBS Maybe SIM amount > OBS amount |
| 2 m b.g.s. | 1. Peak 2. Peak Breakthrough time: Shape/Size: |
1. Peak 2. Peak Breakthrough time: Shape/Size: |
- (SIM ~ OBS Temporarily below GWT- Not used in the calibration procedure) |
- ( SIM ~ OBS Temporarily below GWT- Not used in the calibration procedure) |
- (SIM ~ OBS Temporarily below GWT- Not used in the calibration procedure) |
| - Bromide – monitoring wells at app. 3 m b.g.s. | SIM ~ OBS (GWT 3-4m b.g.s.) |
SIM ~ OBS (GWT 1-3m b.g.s.) |
SIM ~ OBS (GWT 1-3m b.g.s.) |
SIM ~ OBS (GWT 0-4m b.g.s.) |
SIM ~ OBS (GWT 1-3m b.g.s.) |
| - Acc. bromide leaching in drains | - | - | Breakthrough time: () Shape/Size: SIM ~ OBS excluding Jan- May 2004 |
Breakthrough time: Shape/Size: SIM ~ OBS excluding Jan- May 2004 |
Breakthrough time: Shape/Size: SIM ~ OBS since climate series is obtained from station at bit away |
1.1.1 Data
The extensive amount of data collected within the PLAP programme form the basis of the calibration of the scenarios. The following provides an overview of selected data, while detailed information on data acquisition and model set-up are provided by Kjær et al. (2005). For further information on site characterization and monitoring design see Lindhardt et al. (2001).
1.1.1.1 Climate
An automated monitoring system has been installed at each site for measurement of precipitation and barometric pressure. The annual precipitation measured by DIAS and corrected to the soil surface according to the method of Allerup and Madsen (1979) is shown for each site in Table C2.
Table C2. Annual precipitation (mm/year) and potential evapotranspiration (mm/year) at the five sites for the monitoring period.
The potential evapotranspiration has been calculated at DIAS using a modified Makkink equation (Aslyng and Hansen, 1982). The potential evapotranspiration is defined as the evapotranspiration from well-growing short grass adequately supplied with water. The annual potential evapotranspiration at each site is shown in Table 1, and more detailed information on climate data is given in Kjær et al. (2005).
1.1.1.2 Soil
Geological and pedological investigations have been carried out at all sites. Two to three soil profiles have been excavated and described and soil samples have been collected and analyzed. An overview of the soil type at the five sites is given in Table C3.
Table C3. Soil types for the five sites.
| Site | Tylstrup | Jyndevad | Silstrup | Estrup | Faardrup |
| Soil type | Fine sand | Coarse sand | Clayey till | Clayey till | Clayey till |
| Deposited by | Saltwater | Melt water | Glacier | Glacier | Glacier |
For more results on geological and pedological investigations see Lindhardt et al. (2001).
1.1.1.3 Soil Hydrology and Organic Matter
Soil cores (100 cm³ and 6,280 cm³) for the measurement of hydrological properties (soil water characteristics and hydraulic conductivity) have been sampled at three levels corresponding to the A, B and C horizons in the two to three excavated soil profiles at the sites.
The soil water characteristics of the nine small cores (100 cm³) from each horizon are shown together with bulk density, porosity and organic matter in Tables C4 – C8. Measurements of saturated hydraulic conductivity and air permeability using small (100 cm³) or large (6,280 cm³) soil samples at the sites are shown in Figures C3 – C7 corresponding to the three horizons. Additional information on monitoring design and hydraulic data can be found in Lindhardt et al. (2001).
Table C4. Soil water characteristics at Tylstrup determined on the small soil cores, pF = log10(-h). 1) Assuming a particle density of 2.65 g cm-3, 2) Not measured, and 3) Mid-point of the soil core. 4) OM: Organic matter in horizon, OM = 1.72 x TOC. Analysed by DIAS. (Lindhardt et al., 2001)
| Profile no. | Horizon | Depth³ [cm b.g.s.] |
Water content at pF values [cm³ cm-3] |
Bulk density [g cm-3] |
Porosity1 [cm³cm-3] |
OM 4 [%] |
||||||
| 1.0 | 1.2 | 1.7 | 2.0 | 2.2 | 3.0 | 4.2 | ||||||
| 1 (3087) | Ap | 15 | 0.44 | 0.43 | 0.41 | 0.33 | 0.28 | 0.15 | 0.04 | 1.33 | 0.50 | 2.7 |
| Bv | 55 | 0.48 | 0.47 | 0.40 | 0.23 | 0.18 | 0.10 | 0.05 | 1.31 | 0.50 | 2.0 | |
| BC | 80 | 0.45 | 0.44 | 0.41 | 0.24 | 0.16 | 0.07 | 0.01 | 1.40 | 0.47 | 0.3 | |
| 2 (West) | Ap | 15 | 0.42 | 0.41 | 0.41 | 0.36 | 0.28 | 0.16 | 2) | 1.45 | 0.45 | 2) |
| Bh | 60 | 0.44 | 0.42 | 0.33 | 0.19 | 0.15 | 0.10 | 2) | 1.39 | 0.48 | 2) | |
| C | 100 | 0.40 | 0.38 | 0.31 | 0.16 | 0.11 | 0.06 | 2) | 1.51 | 0.43 | 2) | |
| 3 (3088) | Ap1 | 15 | 0.41 | 0.41 | 0.38 | 0.33 | 0.27 | 0.15 | 0.05 | 1.45 | 0.45 | 2.7 |
| Ap2 | 50 | 0.39 | 0.38 | 0.31 | 0.18 | 0.14 | 0.08 | 0.03 | 1.50 | 0.44 | 1.4 | |
| C | 100 | 0.40 | 0.39 | 0.37 | 0.21 | 0.12 | 0.05 | 0.01 | 1.51 | 0.43 | 0.2 | |
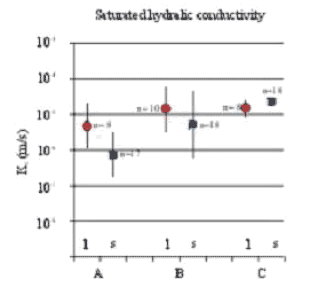
Figure C3. Measured at Tylstrup: Saturated hydraulic conductivity (Ks) measured on large (6,280 cm³) samples (![]() ) and small (100 cm³) samples (
) and small (100 cm³) samples (![]() ). (Lindhardt et al., 2001)
). (Lindhardt et al., 2001)
Table C5. Soil water characteristics at Jyndevad determined on the small soil cores, pF = log10(-h). 1) Assuming a particle density of 2.65 g cm-3, 2) Not measured, and 3) mid-point of the soil core. 4) OM: Organic matter in horizon, OM = 1.72 x TOC. Analysed by DIAS. (Lindhardt et al., 2001)
| Profile no. | Horizon | Depth³ [cm b.g.s.] |
Water content at pF values [cm³ cm-3] |
Bulk density [g cm-3] |
Porosity1 [cm³ cm-3] | OM 4 [%] |
||||||
| 1.0 | 1.2 | 1.7 | 2.0 | 2.2 | 3.0 | 4.2 | ||||||
| 1 (3092) | Ap | 15 | 0.41 | 0.40 | 0.23 | 0.19 | 0.17 | 0.12 | 0.04 | 1.37 | 0.48 | 2.3 |
| Bhs/Bs | 40 | 0.35 | 0.33 | 0.15 | 0.11 | 0.10 | 0.07 | 0.01 | 1.49 | 0.44 | 1.3/0.3 | |
| BC | 115 | 0.34 | 0.32 | 0.10 | 0.06 | 0.06 | 0.04 | 0.01 | 1.52 | 0.43 | 0.1 el. 0.3 | |
| 2 (3091) | Ap | 15 | 0.40 | 0.39 | 0.28 | 0.22 | 0.20 | 0.13 | 0.05 | 1.42 | 0.47 | 3.4 |
| Bhs/Bs | 40 | 0.39 | 0.36 | 0.20 | 0.15 | 0.14 | 0.10 | 0.03 | 1.38 | 0.48 | 1.9/0.5 | |
| C | 130 | 0.34 | 0.28 | 0.09 | 0.07 | 0.06 | 0.05 | 0.01 | 1.48 | 0.44 | 0.1 | |
| 3(North) | Ap | 15 | 0.39 | 0.38 | 0.22 | 0.17 | 0.16 | 0.11 | 0.03 | 1.43 | 0.46 | 2) |
| Bhs | 40 | 0.37 | 0.35 | 0.10 | 0.07 | 0.07 | 0.05 | 0.03 | 1.47 | 0.44 | 2) | |
| C | 110 | 0.34 | 0.32 | 0.09 | 0.06 | 0.05 | 0.04 | 0.01 | 1.50 | 0.43 | 2) | |
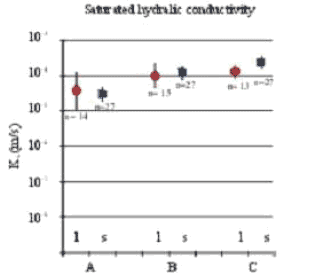
Figure C4. Measured at Jyndevad: Saturated hydraulic conductivity (Ks) measured on large (6,280 cm³) samples (![]() ) and small (100 cm³) samples (
) and small (100 cm³) samples (![]() ). (Lindhardt et al., 2001)
). (Lindhardt et al., 2001)
Table C6. Soil water characteristics at Silstrup determined on the small soil cores, pF = log10(-h). 1) Assuming a particle density of 2.65 g cm-3, 2) Not measured, and 3) mid-point of the soil core. 4) OM: Organic matter in horizon, OM = 1.72 x TOC. Analysed by DIAS. (Lindhardt et al., 2001)
| Profile no. |
Horizon | Depth³ [cm b.g.s.] |
Water content at pF values [cm³ cm-3] |
Bulk density [g cm-3] |
Porosity1 [cm³ cm-3] |
OM 4 [%] |
||||||
| 1.0 | 1.2 | 1.7 | 2.0 | 2.2 | 3.0 | 4.2 | ||||||
| 1 (3093) | Ap | 15 | 0.39 | 0.38 | 0.36 | 0.35 | 0.34 | 0.28 | 0.13 | 1.42 | 0.46 | 3.4 |
| Bv | 40 | 0.35 | 0.35 | 0.32 | 0.31 | 0.30 | 0.25 | 0.16 | 1.62 | 0.39 | 0.5 | |
| BC(g) | 150 | 0.30 | 0.30 | 0.29 | 0.28 | 0.28 | 0.26 | 0.16 | 1.77 | 0.33 | 0.2 | |
| 2 (3094) | Ap | 15 | 0.37 | 0.36 | 0.35 | 0.34 | 0.34 | 0.29 | 0.14 | 1.54 | 0.42 | 2.8 |
| Bv | 40 | 0.36 | 0.36 | 0.35 | 0.34 | 0.33 | 0.29 | 0.15 | 1.59 | 0.40 | 0.5 | |
| Cc | 90 | 0.31 | 0.30 | 0.29 | 0.29 | 0.28 | 0.26 | 0.12 | 1.73 | 0.35 | 2) | |
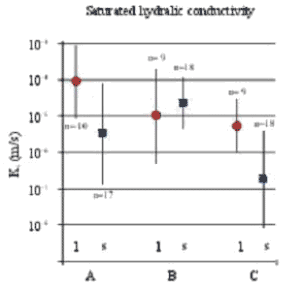
Figure C5. Measured at Silstrup: Saturated hydraulic conductivity (Ks) measured on large (6,280 cm³) samples (![]() ) and small (100 cm³) samples (
) and small (100 cm³) samples (![]() ). (Lindhardt et al., 2001)
). (Lindhardt et al., 2001)
Table C7. Soil water characteristics at Estrup determined on the small soil cores, pF = log10(-h). 1) Assuming a particle density of 2.65 g cm-3, 2) Not measured, and 3) mid-point of the soil core. 4) OM: Organic matter in horizon, OM = 1.72 x TOC. Analysed by DIAS. (Lindhardt et al., 2001)
| Profile no. | Horizon | Depth³ [cm b.g.s.] |
Water content at pF values [cm³ cm-3] |
Bulk density [g cm-3] |
Porosity1 [cm³ cm-3] |
OM 4 [%] |
||||||
| 1.0 | 1.2 | 1.7 | 2.0 | 2.2 | 3.0 | 4.2 | ||||||
| 1 (3099) | Ap | 15 | 0.35 | 0.34 | 0.33 | 0.31 | 0.30 | 0.24 | 0.09 | 1.56 | 0.41 | 2.7 |
| Bt(g) 2 | 36 | 0.33 | 0.32 | 0.31 | 0.30 | 0.29 | 0.26 | 0.20 | 1.73 | 0.35 | 0.2 | |
| Cc 2 | 122 | 0.37 | 0.37 | 0.36 | 0.36 | 0.35 | 0.33 | 0.22 | 1.78 | 0.33 | 0.5 | |
| 2 (3098) | Ap | 15 | 0.37 | 0.36 | 0.34 | 0.33 | 0.33 | 0.27 | 0.13 | 1.56 | 0.41 | 4.2 |
| BE(g) 2 | 50 | 0.38 | 0.38 | 0.37 | 0.37 | 0.37 | 0.33 | 0.06 | 1.67 | 0.37 | 0.5 | |
| 3Cg 2 | 122 | 0.45 | 0.45 | 0.45 | 0.44 | 0.44 | 0.41 | 0.02 | 1.59 | 0.40 | 0.1 | |
| 3 (3100) | Ap | 15 | 0.42 | 0.41 | 0.39 | 0.38 | 0.38 | 0.31 | 0.10 | 1.42 | 0.46 | 5.5 |
| Bhs 2 | 38 | 0.33 | 0.32 | 0.29 | 0.27 | 0.27 | 0.23 | 0.04 | 1.69 | 0.36 | 0.8 | |
| 2C 2 | 120 | 0.42 | 0.42 | 0.42 | 0.41 | 0.40 | 0.37 | 0.31 | 1.55 | 0.42 | 2) | |
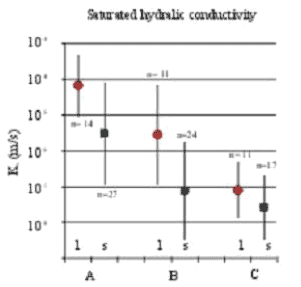
Figure C6. Measured at Estrup: saturated hydraulic conductivity (Ks) measured on large (6,280 cm3) samples (![]() ) and small (100 cm3) samples (
) and small (100 cm3) samples (![]() ). (Lindhardt et al., 2001)
). (Lindhardt et al., 2001)
Table C8. Soil water characteristics at Faardrup determined on the small soil cores, pF = log10(-h). 1) Assuming a particle density of 2.65 g cm-3, 2) Not measured, and 3) mid-point of the soil core. 4) OM: Organic matter in horizon, OM = 1.72 x TOC. Analysed by DIAS. (Lindhardt et al., 2001)
Profile no. |
Horizon | Depth³ [cm b.g.s.] |
Water content at pF values [cm³ cm-3] |
Bulk density [g cm-3] |
Porosity1 [cm3 cm-3] |
OM 4 [%] |
||||||
| 1.0 | 1.2 | 1.7 | 2.0 | 2.2 | 3.0 | 4.2 | ||||||
| 1 (West) | Ap | 15 | 0.37 | 0.36 | 0.32 | 0.30 | 0.28 | 0.21 | 0.08 | 1.42 | 0.46 | 2) |
| Bvt | 75 | 0.32 | 0.31 | 0.28 | 0.26 | 0.25 | 0.20 | 0.13 | 1.60 | 0.40 | 2) | |
| Cc(g) | 120 | 0.27 | 0.27 | 0.25 | 0.23 | 0.23 | 0.21 | 0.10 | 1.84 | 0.31 | 2) | |
| 2(3090) | Ap | 15 | 0.32 | 0.31 | 0.29 | 0.28 | 0.27 | 0.22 | 0.09 | 1.73 | 0.35 | 2.6 |
| Bv | 80 | 0.32 | 0.31 | 0.29 | 0.27 | 0.26 | 0.22 | 0.10 | 1.70 | 0.36 | 0.4 | |
| Cc(g) | 130 | 0.29 | 0.28 | 0.27 | 0.26 | 0.25 | 0.23 | 0.09 | 1.78 | 0.33 | 0.2 - 0.4 | |
| 3(3089) | Ap | 15 | 0.38 | 0.37 | 0.33 | 0.32 | 0.30 | 0.24 | 0.08 | 1.52 | 0.43 | 2.4 |
| Bvt(g) | 80 | 0.32 | 0.30 | 0.27 | 0.25 | 0.24 | 0.20 | 0.15 | 1.70 | 0.36 | 0.2 | |
| Cc(g) | 130 | 0.29 | 0.28 | 0.26 | 0.25 | 0.24 | 0.22 | 0.10 | 1.83 | 0.31 | 0.1 | |
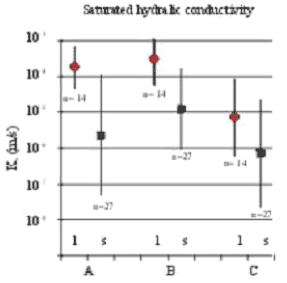
Figure C7. Measured at Faardrup: Saturated hydraulic conductivity (Ks) measured on large (6,280 cm³) samples (![]() ) and small (100 cm³) samples (
) and small (100 cm³) samples (![]() ). (Lindhardt et al., 2001)
). (Lindhardt et al., 2001)
1.1.1.4 Crop
The crops grown at the five sites during the full monitoring period are shown in Table C9.
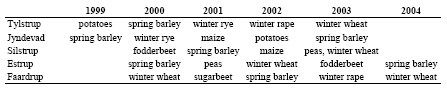
Table C9. Crops grown at the five sites during the monitoring period.
1.1.1.5 Bromide Application
Bromide in a dose of 30 kg/ha has been applied to the five sites at the dates shown in Table C10.
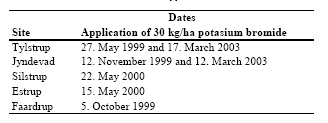
Table C10. Dates of bromide application.
1.1.2 Calibration of Tylstrup Model
The model for the Tylstrup site has been calibrated for the whole period to the observed groundwater table measured in the piezometers located in the buffer zone, to time series of soil water content measured at three different depths (25, 60 and 110 cm b.g.s.) from the two profiles S1 and S2 (see Figure C8) and to the bromide concentration measured in the suction cups located 1 and 2 m b.g.s. The model is evaluated with respect to monitoring measurements of bromide below 3 m b.g.s. Data acquisition and model set-up are described in Kjær et al. (2005) appendix 4. The main calibration parameters were the empirical parameter, BGRAD, which regulates the boundary flow, the “boundary” pressure head (CTEN), its corresponding water content (XMPOR), the hydraulic conductivity (KSM), the dispersivity (DV), the mixing depth (ZMIX) and the effective diffusion path length (ASCALE), which controls the exchange of water and solute between the two flow domains (see Kjær et al. (2005) appendix 4 for details). In addition the solute concentration factor (FSTAR) is calibrated. It accounts for crop uptake of solute in the transpiration stream.
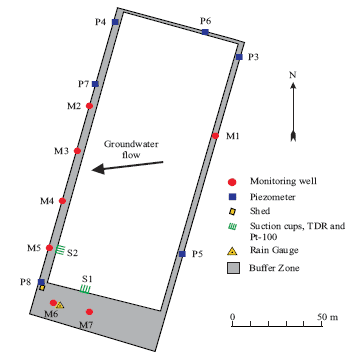
Figure C8. Overview of the Tylstrup test site. The innermost white area indicates the cultivated land, while the grey area indicates the surrounding buffer zone. The positions of the various installations are indicated, as is the direction of groundwater flow (by an arrow). (Kjær et al., 2005)
1.1.3 Soil Water Dynamics and Water Balances
The model simulations are generally consistent with the observed data, thus indicating a good model description of the overall soil water dynamics in the unsaturated zone. The model provides a good simulation of the measured dynamics in the groundwater table (Figure C9-B) but the amplitude of the fluctuations is less well described. The overall trends in soil water content are modelled successfully, with the model capturing soil water dynamics at all depths (Figure C9-C to E). The simulated soil water content in 0.25 and 0.6 m b.g.s. is a little above observed values.
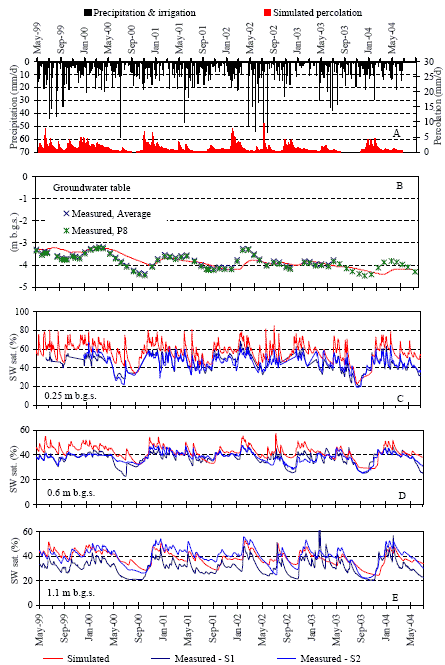
Figure C9. Soil water dynamics at Tylstrup: Measured precipitation, irrigation and simulated percolation 1 m b.g.s. (A), simulated and measured groundwater level (B), and simulated and measured soil water saturation (SW sat.) at three different soil depths (C, D and E). The measured data in B derive from piezometers located in the buffer zone. The measured data in C, D and E derive from TDR probes installed at S1 and S2 (Figure C8).
The resulting annual water balance for Tylstrup is shown for each monitoring period (July–June) in Table C11. For additional information about the water balance in the monitoring period see Kjær et al. (2005).
Table C11. Annual water balance for Tylstrup (mm yr-1). Precipitation is corrected to the soil surface according to the method of Allerup and Madsen (1979). 1) Accumulated for a two-month period, 2) Normal values based on time series for 1961–1990, and 3) Groundwater recharge is calculated as precipitation + irrigation - actual evapotranspiration.
| Period | Normal precipitation 2) |
Precipitation | Irrigation | Actual Evapo-transpiration |
Groundwater recharge 3) |
| 1.5.99–30.6.991) | 120 | 269 | 0 | 112 | 156 |
| 1.7.99–30.6.00 | 773 | 1073 | 33 | 498 | 608 |
| 1.7.00–30.6.01 | 773 | 914 | 75 | 487 | 502 |
| 1.7.01–30.6.02 | 773 | 906 | 80 | 570 | 416 |
| 1.7.02–30.6.03 | 773 | 918 | 23 | 502 | 439 |
| 1.7.03–30.6.04 | 773 | 759 | 0 | 472 | 286 |
1.1.4 Bromide Leaching
Bromide has been applied twice at Tylstrup. In the unsaturated zone the first breakthrough of bromide (deriving from the first application in 1999) is well described by MACRO 5.1 (Figure C10). The dynamics of the second breakthrough of bromide (deriving from the bromide applied in 2003) is less well described by the model (Figure C10). The breakthrough 1 m b.g.s. is simulated two months too early, the concentration increases too rapidly, and the peak is too high compared to the measured profile. At 2 m b.g.s. the simulated concentration decreases too slowly, resulting in a concentration profile that is much wider than the measured profile. Reducing the discrepancies between measured and simulated breakthrough curves in the suction cups was tried by the use of the inverse programme SUFI (Abbaspour et al., 1997) but this was not successful.
Bromide has been measured below the groundwater table in monitoring wells. Because MACRO is a 1-dimensional model it is not correct to calibrate the model to concentrations below the groundwater table. Instead simulated bromide leaching below the groundwater table is evaluated against occurrence of measured bromide at same depths. Measured bromide concentration in the groundwater at 3.5 – 4.5 m b.g.s. in seven wells and simulated bromide concentration at 4 m b.g.s. are shown in Figure C11. The simulated leaching is in agreement with the measured occurrence of bromide.
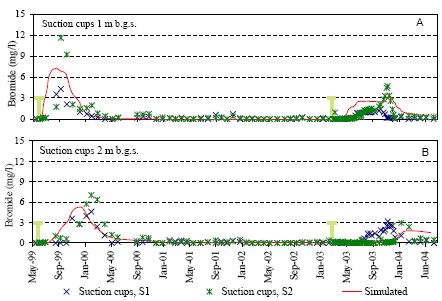
Figure C10. Simulated and measured bromide concentration in the unsaturated zone at Tylstrup: Simulated and measured bromide concentrations at 1 m b.g.s. (A) and 2 m b.g.s. (B). The measured data in A and B derive from suction cups installed 1 m b.g.s. and 2 m b.g.s. at locations S1 and S2 indicated in Figure C8. The green vertical lines indicate the dates of bromide application.
Figure C11. Measured bromide concentration in the groundwater at 3.5 – 4.5 m b.g.s, and simulated bromide concentration at 4 m b.g.s. at Tylstrup. The measured data derive from monitoring wells M1–M7 indicated in Figure C8.
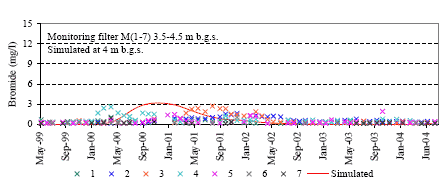
1.1.5 Calibration of Jyndevad Model
The model for the Jyndevad site has been calibrated for the whole monitoring period to the observed groundwater table measured in the piezometers located in the buffer zone, to time series of soil water content measured at three different depths (25, 60 and 110 cm b.g.s.) from the two profiles S1 and S2 (see Figure C12), and to the bromide concentration measured in the suction cups located 1 and 2 m b.g.s. The model is evaluated with respect to monitoring measurements of bromide below 3 m b.g.s. Data acquisition and model set-up are described in Kjær et al. (2005) appendix 4. The main calibration parameters were the empirical parameter BGRAD, which regulates the boundary flow, the “boundary” pressure head (CTEN), its corresponding water content (XMPOR), the hydraulic conductivity (KSM), the dispersivity (DV), the mixing depth (ZMIX) and the effective diffusion path length (ASCALE), which controls the exchange of water and solute between the two flow domains (see Kjær et al. (2005) appendix 4 for details). In addition the solute concentration factor (FSTAR) is calibrated. It accounts for crop uptake of solute in the transpiration stream.
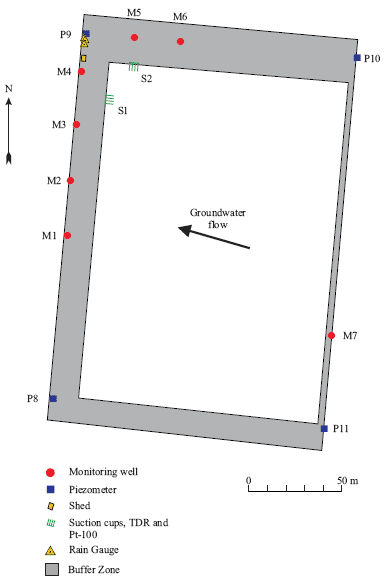
Figure C12. Overview of the Jyndevad test site. The innermost white area indicates the cultivated land, while the grey area indicates the surrounding buffer zone. The positions of the various installations are indicated, as is the direction of groundwater flow (by an arrow). (Kjær et al., 2005).
1.1.6 Soil Water Dynamics and Water Balances
The model simulations are generally consistent with the observed data, thus indicating a good model description of the overall soil water dynamics in the unsaturated zone (Figure C13). The dynamics of the simulated groundwater table is well described. However, as noted in Kjær et al. (2004), the model has some difficulty in capturing the degree of soil water saturation 1.1 m b.g.s. (Figure C13-E).
The resulting annual water balance for Jyndevad for the five monitoring periods (July–June) is shown in Table C12. For additional information about the water balance in the monitoring period see Kjær et al. (2005).
Table C12. Annual water balance for Jyndevad (mm yr-1). Precipitation is corrected to the soil surface according to the method of Allerup and Madsen (1979). 1) Normal values based on time series for 1961–1990, and 2) Groundwater recharge is calculated as precipitation + irrigation - actual evapotranspiration
| Period | Normal precipitation 1) |
Precipitation | Irrigation | Actual Evapo-transpiration |
Groundwater recharge 2) |
| 1.7.99–30.6.00 | 995 | 1073 | 29 | 500 | 602 |
| 1.7.00–30.6.01 | 995 | 810 | 0 | 461 | 349 |
| 1.7.01–30.6.02 | 995 | 1204 | 81 | 545 | 740 |
| 1.7.02–30.6.03 | 995 | 991 | 51 | 415 | 627 |
| 1.7.03–30.6.04 | 995 | 936 | 27 | 429 | 534 |
Figure C13. Soil water dynamics at Jyndevad: Measured precipitation, irrigation and simulated percolation 1 m b.g.s. (A), simulated and measured groundwater level (B), and simulated and measured soil water saturation (SW sat.) at three different soil depths (C, D and E). The measured data in B derive from piezometers located in the buffer zone. The measured data in C, D and E derive from TDR probes installed at S1 and S2 (see Figure C12).
1.1.7 Bromide Leaching
Bromide has been applied twice at Jyndevad. In the unsaturated zone the first breakthrough of bromide (deriving from the first application in 1999) is well described by MACRO 5.1 (Figure C14). The dynamics of the second breakthrough of bromide (deriving from the bromide applied in 2003) is well described by the model in 2 m b.g.s. but less well described in 1 m b.g.s. (Figure C14). At 1 m b.g.s. the second breakthrough is simulated four months too late, and the concentration profile is much narrower than the measured profile. Reducing the discrepancies between measured and simulated breakthrough curves in the suction cups was tried by the use of the inverse programme SUFI (Abbaspour et al., 1997) but this was not successful.
Bromide has been measured below the groundwater table in monitoring wells. Because MACRO is a 1-dimensional model it is not correct to calibrate the model to concentrations below the groundwater table. Instead simulated bromide leaching below the groundwater table is evaluated against occurrence of measured bromide at same depths. Measured bromide concentration in the groundwater at 2.5 – 3.5 m b.g.s. in seven wells and simulated bromide concentration at 3 m b.g.s. are shown in Figure C15. The simulated leaching is in agreement with the measured occurrence of bromide.
Figure C14. Simulated and measured bromide concentration in the unsaturated zone at Jyndevad: Simulated and measured bromide concentrations at 1 m b.g.s. (A) and 2 m b.g.s. (B). The measured data in A and B derive from suction cups installed 1 m b.g.s. and 2 m b.g.s. at locations S1 and S2 indicated in Figure C12. The green vertical lines indicate the dates of bromide application.
Figure C15. Measured bromide concentration in the groundwater at 2.5 – 3.5 m b.g.s, and simulated bromide concentration at 3 m b.g.s. at Jyndevad. The measured data derive from monitoring wells M1–M7 indicated in Figure C12.
1.1.8 Calibration of Silstrup Model
The model for the Silstrup site is calibrated for the whole monitoring period to the observed groundwater table measured in the piezometers located in the buffer zone, to time series of soil water content measured at three depths (25, 60 and 110 cm b.g.s.) from the two profiles S1 and S2 (see Figure C16), to the measured drainage flow, to the bromide concentration measured in the suction cups located 1 and 2 m b.g.s, and to measured bromide leaching in drains. The model is evaluated with respect to monitoring measurements of bromide below 3 m b.g.s. Data acquisition and model set-up are described in Kjær et al. (2005) appendix 4. The main calibration parameters were the empirical parameter BGRAD, which regulates the boundary flow, the “boundary” pressure head (CTEN), its corresponding water content (XMPOR), the hydraulic conductivity (KSM) and the effective diffusion path length (ASCALE), which controls the exchange of water and solute between the two flow domains (see Kjær et al. (2005) appendix 4 for details). In addition the solute concentration factor (FSTAR) is calibrated. It accounts for crop uptake of solute in the transpiration stream.
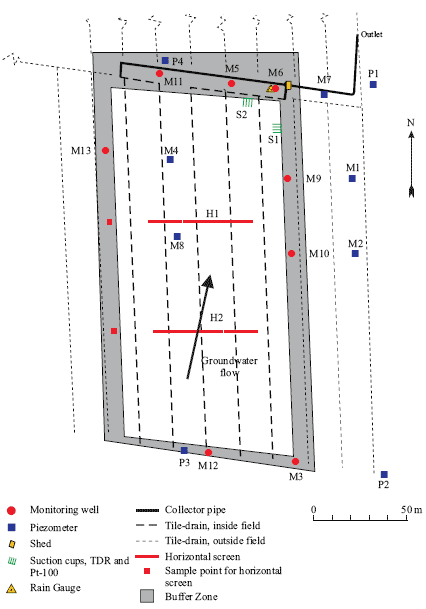
Figure C16. Overview of the Silstrup site. The innermost white area indicates the cultivated land, while the grey area indicates the surrounding buffer zone. The positions of the various installations are indicated, as is the direction of groundwater flow (by an arrow). (Kjær et al., 2005).
1.1.9 Soil Water Dynamics and Water Balances
The model simulations are generally consistent with the observed data, thus indicating a good model description of the overall soil water dynamics in the unsaturated zone (Figure C17). A closer study of measured groundwater table in the different piezometers show that it varies significantly, especially between the upstream (P2 and P3, see Figure C16) and downstream (P1 and P4) piezometers, as shown in Figure C17-B. Calibration to the groundwater table measured in P1 and P4 led to erroneous simulation of drainage flow, which was approximately 200 mm too high for each monitoring year. Calibration to the much more fluctuating groundwater table measured in piezometer P3 yielded a significantly better description of measured drainage. However, the initial rise in the autumn when percolation and drainage flow are initiated is poorly captured. The overall trends in soil water content were described well (Figure C17-D to F).
The resulting annual water balance for Silstrup for the five monitoring periods (July–June) is shown in Table C13. For additional information about the water balance in the monitoring period see Kjær et al. (2005).
Table C13. Annual water balance for Silstrup (mm/year). Precipitation is corrected to the soil surface according to the method of Allerup and Madsen (1979).1)The monitoring was started in April 2000, 2)Normal values based on time series for 1961–1990 corrected to soil surface, 3)Groundwater recharge is calculated as precipitation - actual evapotranspiration - measured drainage, and 4)Where drainage flow measurements are lacking, simulated drainage flow was used to calculate groundwater recharge.
| Period | Normal Precipi- tation 2) |
Precipi-tation | Actual Evapotrans-piration |
Measured drainage | Simulated drainage | Groundwater recharge 3) |
| 1.7.99–30.6.00 1) | 976 | 1175 | 457 | – | 440 | 2774) |
| 1.7.00–30.6.01 | 976 | 909 | 414 | 217 | 230 | 279 |
| 1.7.01–30.6.02 | 976 | 1034 | 470 | 227 | 277 | 337 |
| 1.7.02–30.6.03 | 976 | 879 | 537 | 81 | 72 | 261 |
| 1.7.03–30.6.04 | 976 | 758 | 513 | 148 | 95 | 96 |
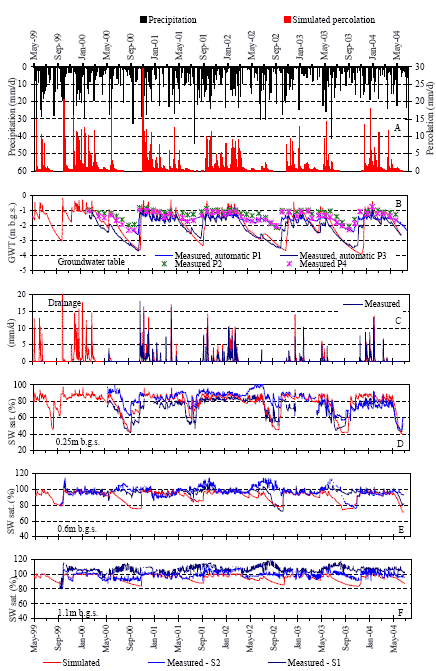
Figure C17. Soil water dynamics at Silstrup: Measured precipitation and simulated percolation 1 m b.g.s. (A), simulated and measured groundwater level (B), simulated and measured drainage flow (C), and simulated and measured soil water saturation (SW sat.) at three different soil depths (D, E and F). The measured data in B derive from piezometers located in the buffer zone. The measured data in D, E and F derive from TDR probes installed at S1 and S2 (see Figure C16).
1.1.10 Bromide Leaching
Bromide has been applied on 22 May 2000 at Silstrup. Two large storm events occurred a few days prior to and after the application of the bromide tracer. The first event caused the onset of a minor flow of drainage water, while the second resulted in rapid percolation and breakthrough of bromide to the drainage system, with the concentration reaching 5.1 mg/l on 29 May (Figure C18-B). When the bromide was applied, the groundwater table was located around 1 m b.g.s. (Figure C17-B). The presence of macropores and the location of the groundwater at the time of bromide application were reflected in the almost instantaneous occurrence of bromide in the drainage water, and suction cups S1 and S2 (Figure C18 and C19). Model simulations of the breakthrough at 1 m b.g.s. are shown in Figure C18-A and at 2 m b.g.s. in Figure C19-A. The dynamics of the breakthrough curves are well described by the model but the breakthrough occurs at the same time as the onset of continuous drainage flow (November 2000). This is about six months later than measured. Reducing the discrepancies between measured and simulated breakthrough curves in the suction cups was tried by the use of the inverse programme SUFI (Abbaspour et al., 1997) but this was not successful.
Bromide leaching to drains is well described (C18-B). Accumulated bromide leaching in the drains is shown in Figure C18-C. The simulated leaching very well corresponds to measured leaching.
Bromide has been measured below the groundwater table in monitoring wells. Because MACRO is a 1-dimensional model it is not correct to calibrate the model to concentrations below the groundwater table. Instead simulated bromide leaching below the groundwater table is evaluated against occurrence of measured bromide at same depths. Measured bromide concentration in the groundwater at 3.5 – 4.5 m b.g.s. in seven wells and simulated bromide concentration at 4 m b.g.s. are shown in Figure C19-B. The simulated leaching is in agreement with the measured occurrence of bromide.
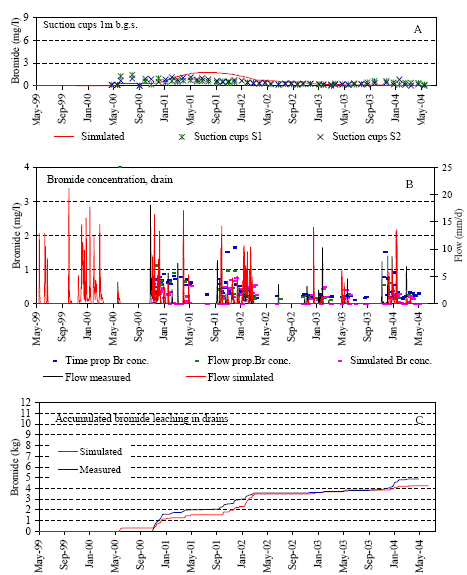
Figure C18. Simulated and measured bromide concentrations above and in the drains at Silstrup. Simulated and measured bromide concentrations at 1 m b.g.s. (A) and in drainage runoff (B). Accumulated simulated and measured bromide leaching in drains (C). The measured data in A derive from suction cups installed 1 m b.g.s. at locations S1 and S2 indicated in Figure C16.
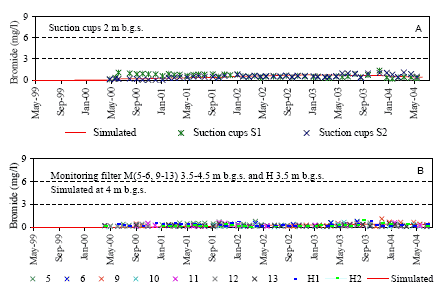
Figure C19. Simulated and measured bromide concentrations below the drains at Silstrup. Simulated and measured bromide concentrations at 2 m b.g.s. (A), measured bromide concentration in the groundwater at 3.5 – 4.5 m b.g.s, and simulated bromide concentration at 4 m b.g.s (B). The measured data in A derive from suction cups installed 2 m b.g.s. at locations S1 and S2 (Figure C16). The measured data in B derive from monitoring wells M5–M13 indicated in Figure C16.
1.1.11 Calibration of Estrup Model
The model for the Estrup site has been calibrated for the whole monitoring period to the observed groundwater table measured in the piezometers located in the buffer zone, to measured drainage flow, to time series of soil water content measured at one depth (25 cm b.g.s.) from a single soil profile S1 (Figure C20), to the bromide concentration measured in the suction cups located 1 and 2 m b.g.s, and to measured bromide leaching in drains. The model is evaluated with respect to monitoring measurements of bromide below 3 m b.g.s. The TDR probes installed at 60 cm and 110 cm b.g.s. yielded unreliable data with saturations far exceeding 100% and dynamics with increasing soil water content during the drier summer periods. No explanation can presently be given for the unreliable data, and they have been excluded from the analysis. The data from the soil profile S2 have also been excluded due to a problem of water ponding above the TDR probes installed at S2, as mentioned in Kjær et al. (2003). Because of the erratic TDR data, calibration data are more limited at this site. Data acquisition and model set-up are described in Kjær et al. (2005) appendix 4. The main calibration parameters were the empirical parameter BGRAD, which regulates the boundary flow, the “boundary” pressure head (CTEN), its corresponding water content (XMPOR), the hydraulic conductivity (KSM) and the effective diffusion path length (ASCALE), which controls the exchange of water and solute between the two flow domains (see Kjær et al. (2005) appendix 4 for details). In addition the solute concentration factor (FSTAR) is calibrated. It accounts for crop uptake of solute in the transpiration stream.
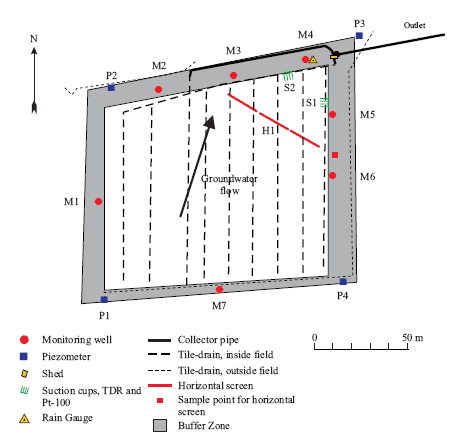
Figure C20. Overview of the Estrup site. The innermost white area indicates the cultivated land, while the grey area indicates the surrounding buffer zone. The positions of the various installations are indicated, as is the direction of groundwater flow (by an arrow). (Kjær et al., 2005)
1.1.12 Soil Water Dynamics and Water Balances
The model simulations are generally consistent with the observed data (which are more limited compared to other PLAP sites, as noted above), indicating a good model description of the overall soil water dynamics in the unsaturated zone (Figure C21). The dynamics of the simulated groundwater table is well described (Figure C21-B) and the model can capture the degree of soil water saturation 0.25 m b.g.s. (Figure C21-D). The simulated drainage (Figure C21-C) matches the measured drainage flow well.
Percolation at Estrup is shown for 0.6 m b.g.s. rather than for 1 m b.g.s. because the soil at 1 m b.g.s. is saturated for longer periods (Figure C21).
The resultant annual water balance for Estrup is shown for the five monitoring periods (July–June) in Table C14. For additional information about the water balance in the monitoring period see Kjær et al. (2005).
Table C14. Annual water balance for Estrup (mm yr-1). Precipitation is corrected to the soil surface according to the method of Allerup and Madsen (1979). 1)Monitoring started in April 2000, 2)Normal values based on time series for 1961–1990 corrected to the soil surface, 3)Groundwater recharge is calculated as precipitation - actual evapotranspiration - measured drainage, and 4) Where drainage flow measurements are lacking, simulated drainage flow was used to calculate groundwater recharge.
| Period | Normal Precipi- tation 2) |
Precipi-tation | Actual evapotrans-piration | Measured drainage | Simulated drainage | Groundwater recharge 3) |
| 1.7.99–30.6.00 1) | 968 | 1173 | 466 | – | 533 | 154 4) |
| 1.7.00–30.6.01 | 968 | 887 | 420 | 356 | 340 | 111 |
| 1.7.01–30.6.02 | 968 | 1290 | 516 | 505 | 555 | 270 |
| 1.7.02–30.6.03 | 968 | 939 | 466 | 329 | 346 | 144 |
| 1.7.03–30.6.04 | 968 | 928 | 496 | 298 | 312 | 134 |
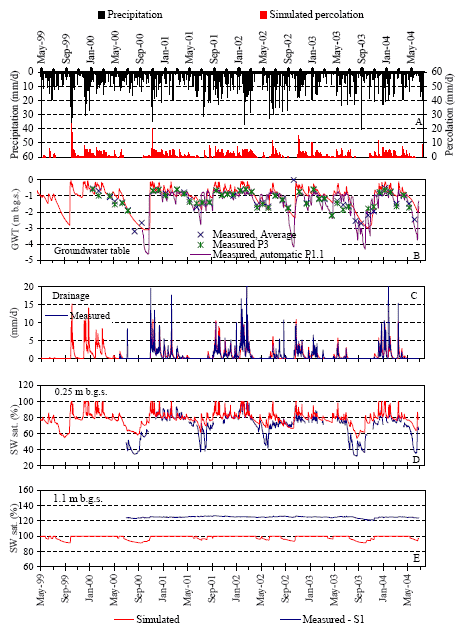
Figure C21. Soil water dynamics at Estrup: Measured precipitation and simulated percolation 0.6 m b.g.s. (A), simulated and measured groundwater level (B), simulated and measured drainage flow (C), and simulated and measured soil saturation (SW sat.) at two different soil depths (D and E). The measured data in B derive from piezometers located in the buffer zone. The measured data in D and E derive from TDR probes installed at S1 (see Figure C20).
1.1.13 Bromide Leaching
Bromide tracer was applied to Estrup in May 2000. Model simulations of the breakthrough at 1 m b.g.s. are shown in Figure C22-A and at 2 m b.g.s. in Figure C23-A. They show that the dynamics of the breakthrough are well described by the model. Simulated concentrations in drainage runoff captures well the measured concentrations (Figure C22-B) and the accumulated simulated bromide leaching in drains is consistent with measured leaching (Figure C22-C).
Bromide has been measured below the groundwater table in monitoring wells. Because MACRO is a 1-dimensional model it is not correct to calibrate the model to concentrations below the groundwater table. Instead simulated bromide leaching below the groundwater table is evaluated against occurrence of measured bromide at same depths. Measured bromide concentration in the groundwater at 3.5 – 4.5 m b.g.s. in seven wells and simulated bromide concentration at 4 m b.g.s. are shown in Figure C23. The simulated leaching is in agreement with the measured occurrence of bromide.
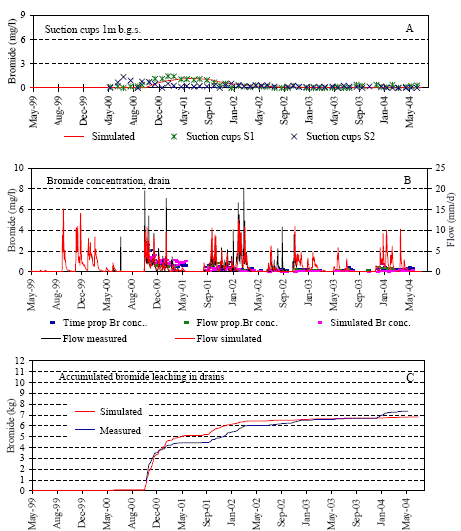
Figure C22. Simulated and measured bromide concentrations above and in the drains at Estrup. Simulated and measured bromide concentrations at 1 m b.g.s. (A) and in drainage runoff (B). Accumulated simulated and measured bromide leaching in drains (C). The measured data in A derive from suction cups installed 1 m b.g.s. at locations S1 and S2 indicated in Figure C20.
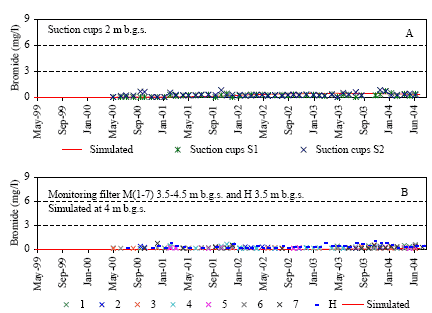
Figure C23. Simulated and measured bromide concentrations below the drains at Estrup. Simulated and measured bromide concentrations at 2 m b.g.s. (A), measured bromide concentration in the groundwater at 3.5 – 4.5 m b.g.s, and simulated bromide concentration at 4 m b.g.s (B). The measured data in A derive from suction cups installed 2 m b.g.s. at locations S1 and S2 (Figure C20). The measured data in B derive from monitoring wells M1–M7 indicated in Figure C20.
1.1.14 Calibration of Faardrup Model
The model for the Faardrup site has been calibrated for the whole monitoring period to the observed groundwater table measured in the piezometers located in the buffer zone, to time series of soil water content measured at three depths (25, 60 and 110 cm b.g.s.) from the two profiles S1 and S2 (Figure C24), to the measured drainage flow, to the bromide concentration measured in the suction cups located 1 and 2 m b.g.s, and to measured bromide leaching in drains. The model is evaluated with respect to monitoring measurements of bromide below 3 m b.g.s. Data acquisition and model set-up are described in Kjær et al. (2005) appendix 4. The main calibration parameters were the empirical parameter BGRAD, which regulates the boundary flow, the “boundary” pressure head (CTEN), its corresponding water content (XMPOR), the hydraulic conductivity (KSM) and the effective diffusion path length (ASCALE), which controls the exchange of water and solute between the two flow domains (see Kjær et al. (2005) appendix 4 for details). In addition the solute concentration factor (FSTAR) is calibrated. It accounts for crop uptake of solute in the transpiration stream.
As stated in Kjær et al. (2003), precipitation measured at Flakkebjerg 3 km east of Faardrup was used for the monitoring periods July 1999–June 2002 due to an electronic noise problem in the automated monitoring system at Faardrup.
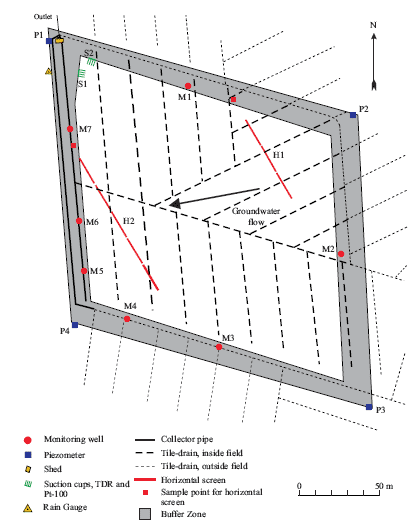
Figure C24. Overview of the Faardrup site. The innermost white area indicates the cultivated land, while the grey area indicates the surrounding buffer zone. The positions of the various installations are indicated, as is the direction of groundwater flow (by an arrow). (Kjær et al., 2005)
1.1.15 Soil Water Dynamics and Water Balances
The model simulations are generally consistent with the observed data, thus indicating a good model description of the overall soil water dynamics in the unsaturated zone (Figure C25). The dynamics and level of the measured groundwater table are well described by the present model. However, the drop in measured groundwater table during the dry summer periods is not fully reflected in the simulations. Furthermore, the measured quick rise in groundwater table after the summer period is too slow in the simulation. The level and dynamics of the soil water content in all three horizons are well described by the model (Figure C25-D, E and F).
The simulated drainage flow closely matches the measured drainage flow (Figure C25-C). However, the simulated peak at the onset of the drainage flow in the monitoring period is less well described. This is probably attributable to the above-mentioned problems with the groundwater table.
The resultant annual water balance for Faardrup is shown for the five monitoring periods (July–June) in Table C15. For additional information about the water balance in the monitoring period see Kjær et al. (2005).
Table C15. Annual water balance for Faardrup (mm yr-1). Precipitation is corrected to the soil surface according to the method of Allerup and Madsen (1979). 1) Normal values based on time series for 1961–1990, 2) For 1.7.99–30.6.02, measured at the DIAS Flakkebjerg meteorological station located 3 km from the test site (see text), and 3) Groundwater recharge is calculated as precipitation - actual evapotranspiration - measured drainage.
| Period | Normal Precipi- tation 1) |
Precipi- tation 2) |
Actual Evapotrans- piration |
Measured drainage | Simulated drainage | Groundwater recharge 3) |
| 1.7.99–30.6.00 | 626 | 715 | 572 | 192 | 147 | -50 |
| 1.7.00–30.6.01 | 626 | 639 | 383 | 50 | 31 | 206 |
| 1.7.01–30.6.02 | 626 | 810 | 531 | 197 | 169 | 85 |
| 1.7.02–30.6.03 | 626 | 633 | 483 | 49 | 75 | 102 |
| 1.7.03–30.6.04 | 626 | 587 | 435 | 36 | 0 | 116 |
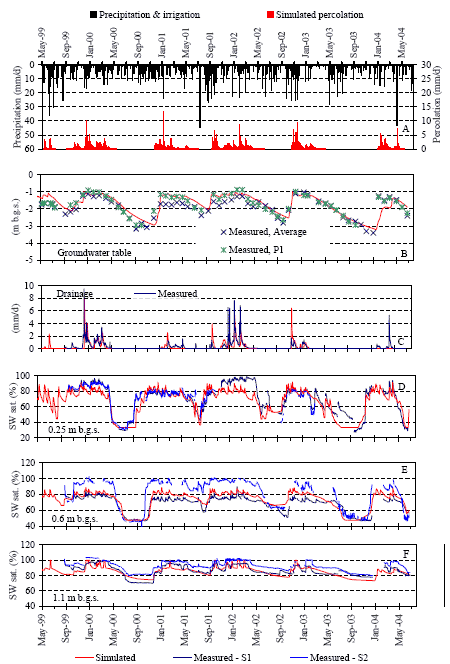
Figure C25. Soil water dynamics at Faardrup: Measured precipitation and simulated percolation 1 m b.g.s. (A), simulated and measured groundwater level (B), simulated and measured drainage flow (C) and simulated and measured soil water saturation (SW sat.) at three different soil depths (D, E, and F). The measured data in B derive from piezometers located in the buffer zone. The measured data in D, E and F derive from TDR probes installed at S1 and S2 (see Figure C24).
1.1.16 Bromide Leaching
Bromide tracer was applied to Faardrup in September 1999. Measured breakthrough curves of bromide to the drainage system 1 m b.g.s. in suction cup S1, and 2 m b.g.s. in suction cup S2 are shown in Figures C26-A and C27-A. Noting that the precipitation is not locally measured for all years, as stated previously, model simulations are able to replicate the breakthrough (Figures C26-A and C27-A). Simulated concentrations in drainage runoff captures well the measured concentrations (Figure C26-B) and the accumulated simulated bromide leaching in drains is almost consistent with measured leaching (Figure C26-C).
Bromide has been measured below the groundwater table in monitoring wells. Because MACRO is a 1-dimensional model it is not correct to calibrate the model to concentrations below the groundwater table. Instead simulated bromide leaching below the groundwater table is evaluated against occurrence of measured bromide at same depths. Measured bromide concentration in the groundwater at 3.5 – 4.5 m b.g.s. in seven wells and simulated bromide concentration at 4 m b.g.s. are shown in Figure C27-B. The simulated leaching is in agreement with the measured occurrence of bromide.
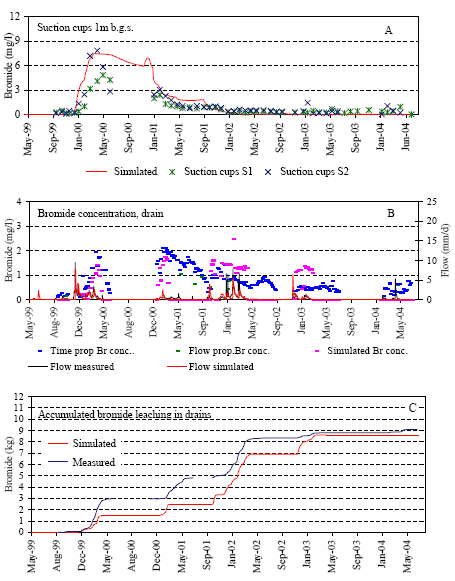
Figure C26. Simulated and measured bromide concentrations above and in the drains at Faardrup. Simulated and measured bromide concentrations at 1 m b.g.s. (A) and in drainage runoff (B). Accumulated simulated and measured bromide leaching in drains (C). The measured data in A derive from suction cups installed 1 m b.g.s. at locations S1 and S2 indicated in Figure C24.
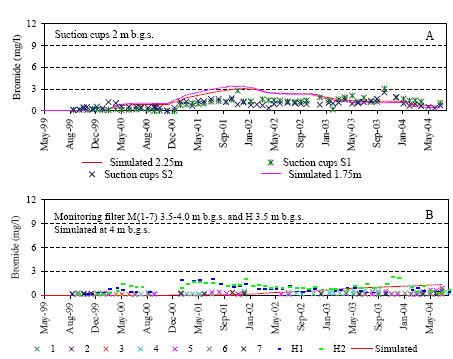
Figure C27. Simulated and measured bromide concentrations below the drains at Faardrup. Simulated and measured bromide concentrations at 2 m b.g.s. (A), measured bromide concentration in the groundwater at 3.5 – 4.5 m b.g.s and simulated bromide concentration at 4 m b.g.s (B). The measured data in A derive from suction cups installed 2 m b.g.s. at locations S1 and S2 (Figure C24). The measured data in B derive from monitoring wells M1–M7 indicated in Figure C24.
Appendix D Modelling Results
This Appendix presents the simulated yearly results of the Karup, Langvad, Hamburg, and the five PLAP-scenarios.
1.1 Karup and Langvad Scenarios
The leaching of Pesticide A, B, and C were simulated using the not official Karup and Langvad FOCUS-scenarios, which are set up with the model MACRO 4.4.2. (http://viso.ei.jrc.it/focus/gw/). The simulated average flux concentration at 1 m b.g.s. for pesticides A, B, and C for respectively the spring and fall application are presented in Figures D1 and D2. The mass balance for Pesticides A, B, and C given respectively spring and fall application for the Karup and Langvad scenarios is shown by MACRO 4.2.2. standard output in Figure D3.
The results show that over a period of 20 years application of pesticide A, B and C in connection with growing of spring or winter cereals, the maximum allowed concentration MAC (0.1 mg/l) is exceeded except for the combination Karup, Winter, Pesticide C; Karup, Spring, Pesticide B; and Karup, Spring, Pesticide C.
In general higher leaching is observed at Langvad than Karup, which is expected to be the opposite because sand should be more permeable than clay. By comparing Table A3 with A5 (Appendix A), a lower saturated hydraulic conductivity at Karup compared to Langvad, could give an explanation.
The level of leaching when growing spring cereals instead of winter cereals is generally lower for pesticide A and C, but for pesticide B (with the smallest DT50 and Koc) the opposite is the case. The MACRO-simulation results seem to indicate that pesticides with short half life and weak sorption properties pose a higher leaching risk under spring application than fall application whereas the opposite is the case for pesticides with long half life and strong sorption properties.
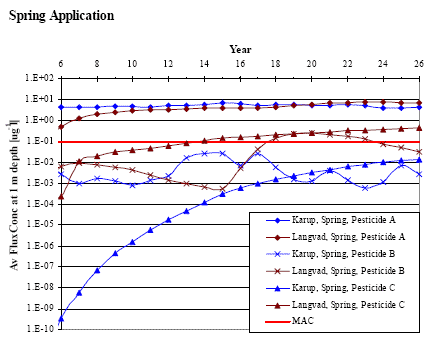
Figure D1. Average flux concentration at 1 m depth of Pesticide A, B, and C given spring application at Karup and Langvad and the maximum allowed concentration (MAC).
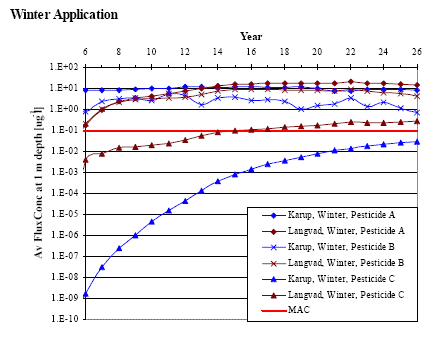
Figure D2. Average flux concentration at 1 m depth of Pesticide A, B, and C given fall application at Karup and Langvad and the maximum allowed concentration (MAC).
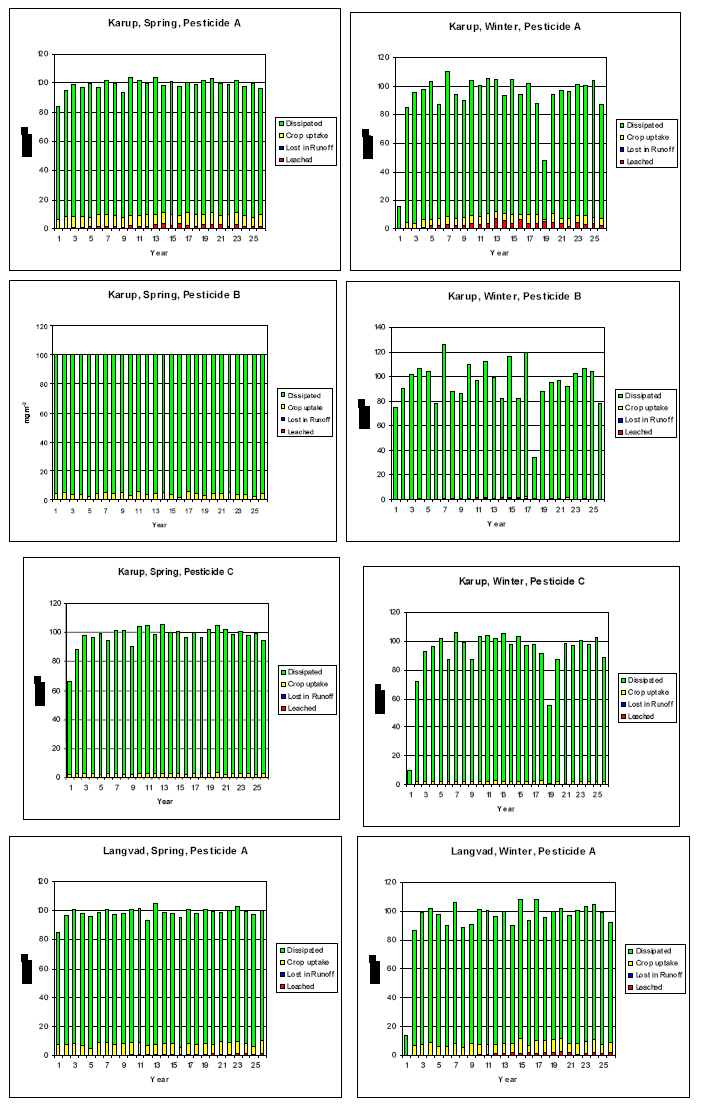
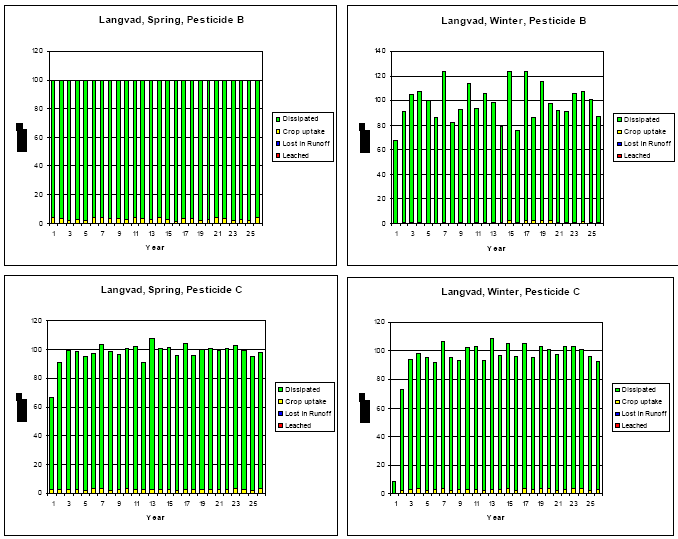
Figure D3. Mass Balance diagrams including dissipated, crop uptake, lost in runoff, and leached for Pesticide A, B, and C given respectively winter and spring application for the Karup and Langvad scenarios.
1.2 Hamburg Scenario
The leaching of Pesticides A, B, and C were simulated using FOCUS-scenario, Hamburg, which is set up with the model PELMO (http://viso.ei.jrc.it/focus/gw/). The simulated average flux concentration at 1 m b.g.s. and at the soil bottom for pesticide A for respectively the spring and fall application are presented with the output of the PELMO model in Figures D4 and D5. For Pesticides B and C they are shown in Figures D6 – D9. In Figures D4 – D9 also the annual pesticide balance for all pesticides are shown.
The results show that over a period of 20 years fall application of pesticide A, B and C causes higher leaching at both 1 m b.g.s and at the bottom of the soil than spring application. Simulated leaching is highest for Pesticide A and smallest for Pesticide C and is often exceeding the maximum allowed concentration MAC (0.1 μg/l). Plant uptake of pesticide is notably larger for Pesticide A than B and C.
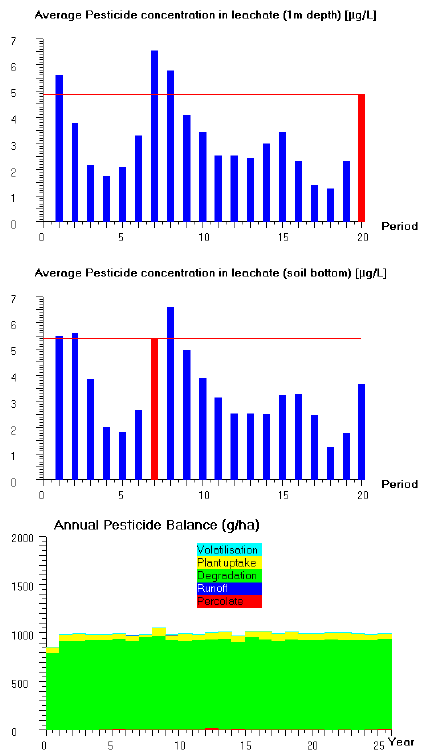
Figure D4. Simulated average flux concentration at 1 m b.g.s. and at the soil bottom for pesticide A for spring cereal with application date April 23. Also the annual pesticide balance including volatilisation, plant uptake, degradation, runofff, and percolate.
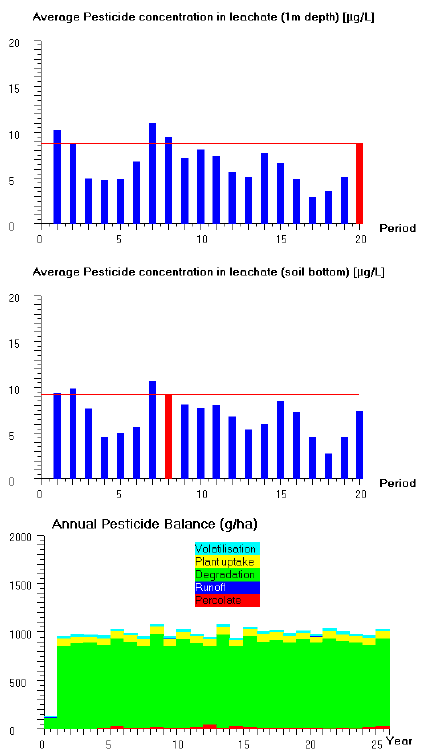
Figure D5. Simulated average flux concentration at 1 m b.g.s. and at the soil bottom for pesticide A for winter cereal with application date November 20. Also the annual pesticide balance including volatilisation, plant uptake, degradation, runofff, and percolate.
Figure D6. Simulated average flux concentration at 1 m b.g.s. and at the soil bottom for pesticide B for spring cereal with application date April 23. Also the annual pesticide balance including volatilisation, plant uptake, degradation, runofff, and percolate.
Figure D7. Simulated average flux concentration at 1 m b.g.s. and at the soil bottom for pesticide B for winter cereal with application date November 20. Also the annual pesticide balance including volatilisation, plant uptake, degradation, runofff, and percolate.
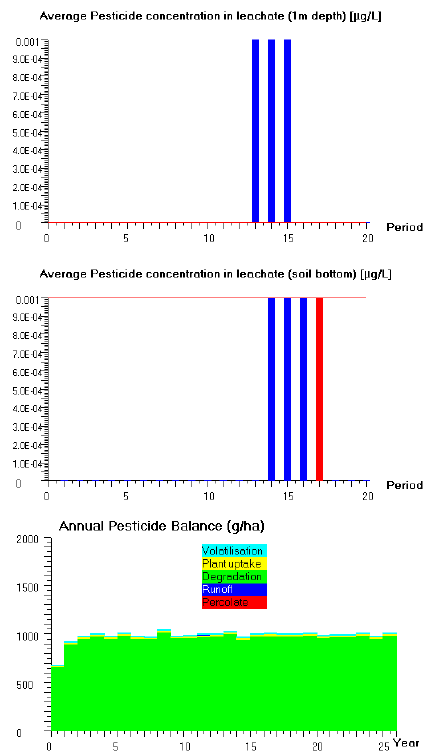
Figure D8. Simulated average flux concentration at 1 m b.g.s. and at the soil bottom for pesticide C for spring cereal with application date April 23. Also the annual pesticide balance including volatilisation, plant uptake, degradation, runofff, and percolate.
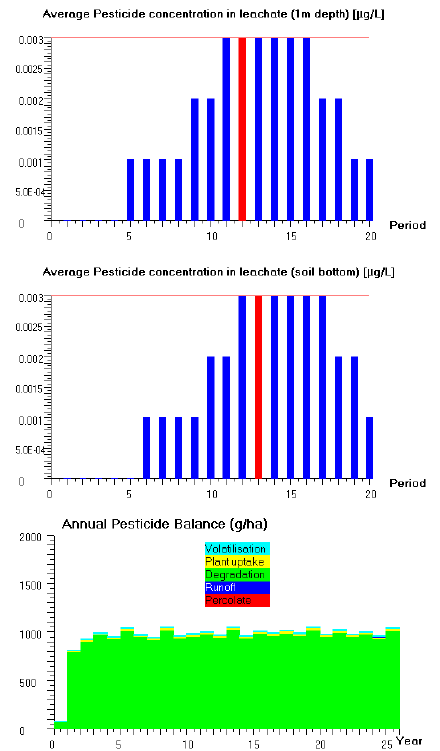
Figure D9. Simulated average flux concentration at 1 m b.g.s. and at the soil bottom for pesticide C for winter cereal with application date November 20. Also the annual pesticide balance including volatilisation, plant uptake, degradation, runofff, and percolate.
1.3 PLAP Scenarios
The leaching of Pesticide A, B, and C were simulated using the calibrated PLAP-scenarios described in Appendix C, which are set up with the model MACRO 5.1.
20 years simulation results of:
- the five PLAP-scenarios (Tylstrup, Jyndevad, Estrup, Silstrup, and Faardrup),
- implementing either the crop Spring cereal or Winter cereal,
- applying either Pesticide A, B or C,
are presented in the following thirty tables including:
- Water Balance (Precipitation+Irrigation, Actual Evaporation, Runoff and Percolate)
- Pesticide Leachate (Leachate at 1 and 3 m b.g.s.)
- Mass Balance (Leaching, Runoff, Degradation, Plant uptake, and Storage + Solute infiltration.
The results show that over a period of 20 years fall application of pesticide A, B and C causes higher leaching than spring application at both 1 m and 3 m b.g.s. Simulated leaching is highest for Pesticide A and is often exceeding the maximum allowed concentration MAC (0.1 μg/l).
Results also show that years of high or low leaching for a scenario are not the same for the three pesticides. The variation in leaching between different years for same scenario, crop and pesticide can be of orders of magnitudes.
In general the greatest leaching at both depths is observed at the clay location Silstrup. Among the two sand locations most leaching is simulated at Jyndevad.
Click here to see Tables.Appendix E 1 Present Use of Model Scenarios
This appendix has been written by Steen Marcher and Claus Hansen from the Danish EPA.
The appendix presents the evolution from the first registration procedure including model scenarios in Denmark up to the present procedure. It also explains the background for using 1 m below ground surface (b.g.s) as reference for decisions on pesticide approval when applying mathematical models.
1.1 Original registration procedure and changes to original procedure
(Written by the Danish EPA)
A decade ago the Danish Environmental Protection Agency (hereafter Danish EPA) did not accept mathematical modelling concepts as adequate documentation on leaching of pesticides to ground water because the current models were not satisfactorily validated and were not specific for Danish conditions regarding soil and climate. By experience from national research and by joining the FOCUS group under the European Commission (FOCUS (1995), the Danish EPA elaborated the first guidance papers on how to evaluate model studies regarding leaching of pesticides and their metabolites to ground water.
The guidance paper was published November 28, 1997 (cited below).The guidance paper was – and still is – a dynamic paper, which will be updated as the administrative process is refined, caused by progress on the modelling and scenario area.
__________________________________________________________________
The first Guidance Paper for Using Mathematical Models in an Assessment of Pesticide Mobility, released November 28, 1997 (Miljøstyrelsen, 1997) - Guidance Paper for Using Mathematical Models in an Assessment of Pesticide Mobility
Object
This paper is intended to describe how the Danish Environmental Protection Agency will use results from mathematical models in the assessment of whether or not the use of a pesticide presents a risk of groundwater contamination and to describe the Agency’s requirements to models, scenarios and input data.
Background
In a number of cases, ordinary documentation in the form of laboratory tests is not sufficient to evaluate the risk of groundwater contamination, nor does supplemental documentation in the form of lysimeter studies always provide a definitive description of the actual risk in the field.
Using models to describe transport and the degradation of pesticides in soil allows testing of many more combinations of factors such as soil type, climatic conditions, application times, anticipated soil exposure, etc. than is otherwise financially and technically possible in lysimeter and field testing.
Modelling is increasingly being used as supplemental documentation. For example, modelling is typically included in EU monographies, and several member countries use models on a national level. The Agency has also in some cases commissioned model runs concerning mobility of pesticides and degradation products.
Since no validated regional EU models for groundwater studies exist as yet, this paper specifies the guidance for the Agency’s requirements to modelling and the evaluation of modelling, which are to apply until common guidelines have been set up for the use of models in the EU.
General Status of Modelling of Pesticide Leaching Today
Constructing and running models of water balances and the transport of conservative compounds is often satisfactory in the case of thoroughly studied scenarios. There are at present no validated models for pesticide transport in Danish conditions, and work on more detailed descriptions of various model parameters - especially the variability in soil conditions - should continue with respect to choice of scenarios. Modelling does not provide exact results, but the results can be indicative and used for comparisons among various compounds and scenarios.
To ensure that model results mirror reality as closely as possible, it is necessary at least to calibrate/validate all desired combinations of soil/climate scenarios against water balances and conservative transport measurement data. The optimal solution would be to use the validation procedure described in the FOCUS report “Leaching models and EU registration”, which includes both lysimeter and field testing. Since such data sets do not currently exist, it might in the short term be possible to validate against existing lysimeter testing data. This would at least ensure that the physical description of soil type is realistic. Realistic boundary conditions such as fluctuating groundwater tables and draining could then be transferred after a calibration against what we know from experience are realistic water balances. Validation against lysimeter data only should, however, in the longer term be supplemented with validation against field data.
There are many uncertainties, e.g. choice of localities (combination of soil type and climate data), choice of substance-specific parameters and, of course, the model’s handling of the various processes of the substance in question. Utilisation of a wide variety of set-up’s and input data will reduce uncertainty - and contain worst-case scenarios too.
Agency Guidance
The Agency has selected two actual localities in Denmark: Langvad and Karup. These localities were chosen, because in connection with the NPO project they were well-described with respect to climate and soil types. Data also exists in the form of field measurements of water balances. On this basis, a regional computer model (MIKE-SHE) was set up which has been validated as far as water balance is concerned. This model was then used to validate the pesticide leaching model MACRO’s water balance at the two localities.
The two scenarios, Karup (light soil with coarse sand) and Langvad (loam featuring many macropores and a rapid downward water transport), are also soils considered to represent a greater risk of leaching than the average of the two soil types found most often in Denmark: sandy soil and loam. Also, both localities are in areas receiving more precipitation than is average for either sandy soil or loam. Thus Karup and Langvad are considered to represent realistic worst-case situations.
Climatic variation is to be handled by using long consecutive time series with annual applications. Critical precipitation events are included by simulated dosing every single day of the time period in which the applicant specifies the pesticide is to be used.
The variation in substance-specific parameters - degradation and sorption - can be described using sensitivity analysis (sensitivity runs of the model) or, alternatively, by choosing the least favourable combination of degradation rate and sorption conditions.
After discussion with, among others, GEUS (Geological Survey of Denmark and Greenland) and the Danish Hydraulic Institute, and taking into account results and recommendations from, among others, the EU’s modelling work group FOCUS and the Agency’s Pesticide Research Program, the following guidance for models, scenarios, input data and interpretation of results is set up.
Modelling and Scenarios
- Models: A model code should be used in which it is possible to incorporate preferential transport, including macropore and capillary flow. The model must also be applicable to Danish conditions, which at this time means using the MACRO or MIKE-SHE models.
- If other model codes - or new versions of existing model codes - are used, then reporting must document that the calibrated water balance corresponds to the scenarios previously run.
- Soil types and localities: The soils/localities specified by the Agency must be used, which at this time means two typical Danish soils representing sandy soil (Karup) and till with preferential flow (Langvad).
- Climate data: Long series of time must be used, i.e. 30 and 24 years respectively for the two above-mentioned localities.
- Substance-specific parameters: A realistic worst-case combination of degradation rate and sorption conditions is to be chosen, e.g. on the basis of a sensitivity analysis.
- Application: Application of the highest dose specified by the applicant should be used in the model. Separate model runs must be made for each day of the period of time during which the applicant specifies the pesticide is to be used. If this time period is very long, however, dividing it into segments may be considered.
- Crops: If several crops are used, the worst-case crop with respect to plant coverage, root development, etc. should be selected if possible. Alternatively, runs can be made for all crops.
- Results must be stated as annual or seasonal averages. Peak values should also be listed.
- If other values/input data than those specified by the Agency/default values are used, then an explanation of why must be included.
Interpretation of Results
- The assessment will be based on the average amount, which leaches down below the root zone (at a depth of about one metre) per year or season in the individual runs.
- The number of instances in which amount leached exceeds the maximum residue limit is to be compared to the total number of runs. If the number of such instances exceeds a certain specified total number of instances, then the use applied for cannot be approved on the basis of the modelling performed.
The above-mentioned specifications are guiding only. An ad hoc evaluation must always be made for each pesticide on the basis of all the material available: laboratory, lysimeter and field testing as well as monitoring, modelling, etc.
The guidance stated here will be modified as new data and know-how become available.
User guidance
The Agency will set up certain requirements, including a detailed description of scenarios (soil types, water balances, climate data, etc.) and accompanying documentation of the calibrated water balances used previously for Karup and Langvad. These water balances must be reproducible and are to be documented by reporting model results.
The Agency offered this data on CD-ROM under certain conditions by the end of 1997.
End of Guidance Paper
Some comments can be made on the first Guidance paper:
The scenarios Karup and Langvad were chosen simply because it was the best described scenarios, probably the only useable, and because they at that time was assessed as the most representative realistic worst case scenarios for Danish conditions.
The reason to choose 24 years and 30 years for the two scenarios, respectively, was that this was the existing time periods.
By experience and progress in the model concept the guidance paper from 1997 was refined and clarified in the Danish EPA frameworks for assessment of pesticides 28 May 1999 (cited below).
The major progresses were as follows:
It was now no longer necessary to model application on every single day for long application periods. Instead representative sub periods could be accepted.
The results should alone be reported as annual averages. The Danish EPA no longer found reasons for requiring seasonal averages and peak values as only the annual averages should be evaluated.
A more explicit formulation of the trigger for safe use regarding ground water was presented: To support approval for the proposed application the limit value of 0.1 μg/L must not exceed 5 % of the occasions meaning that the 95th percentile regarding the output data should be used.
__________________________________________________________________
The second Guidance Paper for Using Mathematical Models in an Assessment of Pesticide Mobility, released May 28, 1999 - Evaluation of mathematical modelling of risk of ground water pollution (Miljøstyrelsen, 1999)
The leaching of active ingredients and metabolites will usually be assessed with respect to the substance’s intrinsic properties, lysimeter tests or field studies. Unless the results irrefutably show that no unacceptable leaching will take place under Danish conditions, mathematical modelling must be carried out and included in the overall assessment.
As validated regional ground water models still not excist in the EU the Danish Environmental Protection Agency has made a guidance paper for mathematical modelling of the risk of ground water pollution from plant protection products. To use the modelling in the evaluation the mentioned guidance instructions should be followed. Alternatively, deviations from the guidance instructions should be justified and/or “worst case” situations should be used.
The following requirements for modelling and scenarios must be satisfied:
§ Models: A model code, which can indicate preferential transport mechanisms, including macropore flow and capillary rise, must be used. The model shall be usable for Danish conditions. This means the “MACRO” and “MIKE-SHE” models. If another model code is used, the report must document the way in which the calibrated water balance corresponds to the Danish scenarios.
§ Soil types and localities: The soils/localities specified by the Danish EPA are used – at present, two typical Danish soils, representing sandy soil (Karup) and moraine clay with preferential flow (Langvad).
§ Climate data: Time series over 30 and 24 years, respectively, for Karup and Langvad must be used.
§ Substance-specific parameters: A realistic “worst case” combination of degradation rate and sorption, ex. based on sensitivity analyse, must be used.
§ Crop: Where several crops are involved, the worst-case crop (with respect to vegetation mantle, root development, etc.) must be used where possible. Alternatively, all crops must be modelled.
§ Application: Application of the highest dose for which approval is sought must be modelled. Separate model runs should be presented for every single day in the application period. Alternatively, if the application period is very long, representative sub periods can be accepted.
§ The results must be reported as annual averages.
§ All values/input other than those set by the Danish EPA/default values must be justified.
The appraisal is done on the basis of the average annual leaching to below the root zone (a depth of about one meter). The number of occasions when leaching exceeds the limit values are accounted against the total number of runs. If the limit is exceeded on more than a specified proportion of the occasions (5%, as the point of departure), the model runs cannot be used to support approval for the proposed application.
End of Guidance Paper
The following years the practical experience of the Danish EPA in assessing approvals on pesticides showed that practically no pesticides or metabolites could be forced to leach through the Langvad moraine clay scenario by preferential flow when using the above mentioned model set-up. This modelling result was in contrast to monitoring results showing that pesticides and their metabolites in some cases depending on compound and uses could be detected under moraine soils. Moreover, GEUS concluded that the Langvad scenario was not a worst case scenario regarding Danish moraine clay soils. Therefore, the Danish EPA found that it did not make sense still to require modelling on the Langvad scenario. For this reason the Danish EPA considered to accept modelling alone on the Karup scenario.
Another experience was that the Karup sandy soil scenario was not as conservative as expected comparing the model results with the FOCUS PELMO Hamburg scenario. Actually, the results showed a comparable or even a little higher leaching in Hamburg than in Karup. At the same time GEUS [står dette i den første VAP rapport?] confirmed that the Karup scenario was not a worst case scenario for Danish conditions. In this light it seemed reasonable to accept modelling on the PELMO Hamburg scenario in stead of using the two Danish scenarios: Langvad and Karup.
Moreover, again learning by experience it seemed to be too conservative to require a worst case combination of degradation rate and sorption parameters. In practise, the possibility that the highest degradation rate would coincide with med the lowest adsorption coefficient was very low. Therefore, the requirement was changed to use 80th percentile values for degradation rate and sorption conditions (including 1/n) derived from studies representative for Danish conditions. Compared with the recommendation from EU FOCUS ground water modelling of using 50th percentile values for the two parameters the Danish guidance was still relatively conservative.
Finally, the Danish EPA found that it was not necessary to require modelling for every single day in the theoretical application period.
On this background the Danish EPA of practical reasons refined the requirement as described in an updated annex to the frameworks for assessment of pesticides June 21, 2005.
__________________________________________________________________
The third Guidance Paper for Using Mathematical Models in an Assessment of Pesticide Mobility, released June 21, 2005 - Framework for the assessment of plant protection products (Miljøstyrelsen, 2005)
Appraisal of mathematical modelling on risk of ground water pollution
The leaching of active ingredients and metabolites will usually be assessed with respect to the substance’s intrinsic properties, lysimeter tests or field studies. Unless the results irrefutably show that no unacceptable leaching will take place under Danish conditions, mathematical modelling must be carried out and included in the overall assessment.
The following requirements for modelling and scenarios must be satisfied:
§ Models: A model code, which can indicate preferential transport mechanisms, including macropore flow and capillary rise, must be used. The model shall be usable for Danish conditions. This means the “MACRO” and “MIKE-SHE” models. If another model code is used, the report must document the way in which the calibrated water balance corresponds to the Danish scenarios. Alternatively the PELMO model and the Hamburg scenario from FOCUS can be applied.
§ Soil types and localities: The soils/localities specified by the Danish EPA are used – at present, two typical Danish soils, representing sandy soil (Karup) and moraine clay with preferential flow (Langvad) or the Hamburg scenario from FOCUS
§ Climate data: Time series over 30 and 24 years, respectively, for Karup and Langvad must be used and 20 years (+ 6 years calibration) for Hamburg.
§ Substance-specific parameters: 80th percentile values must be used for degradation rate and sorption conditions (including 1/n). These values must be derived from studies that are relevant/representative for Danish conditions.
§ Crop: Where several crops are involved, the worst-case crop (with respect to vegetation mantle, root development, etc.) must be used where possible. Alternatively, all crops must be modelled.
§ Application: Application of the highest dose for which approval is sought must be modelled. To illustrate the sensitivity to changing the date of application separate model runs should be presented for different dates during the period in which use of the product is proposed.
§ The results must be reported as annual averages.
§ All values/input other than those set by the Danish EPA/default values must be justified.
The appraisal is done on the basis of the average annual leaching to below the root zone (a depth of about one meter). The number of occasions when leaching exceeds the limit values are accounted against the total number of runs. If the limit is exceeded on more than a specified proportion of the occasions (5%, as the point of departure), the model runs cannot be used to support approval for the proposed application.
End of Guidance Paper
Caused by this revised Guidance paper it was now possible for the applicants when applying for approval of pesticides in Denmark to use the results from the EU registration procedure (the FOCUS PELMO on the Hamburg scenario) with only two corrections: Use of the 80th percentile in stead of the geometric mean for degradation rate and sorption constant, and use of the 95th percentile in stead of the 80th percentile regarding the output data (average leaching in the 1 metres reading point).
The applicants were very satisfied by the possibility to use the FOCUS PELMO on the Hamburg scenario. Actually, the Danish EPA have not received modelling on the Danish scenarios Karup and Langvad after releasing the above mentioned revised Guidance Paper.
__________________________________________________________________
1.2 The Professional Administrative Rationale for using Pesticide Leaching at 1m in the Registration Procedure
(Written by the Danish EPA)
1.2.1 The Danish Approach
The selection of 1 metre as a reading point when assessing potential ground water pollution with pesticides and their metabolites has both practical and historical reasons.
One and a half decade ago the Danish EPA (Environmental Protection Agency) decided to set up criteria for the acceptance of pesticides. A working group with delegates from the Pesticide Advisory Board and the Danish EPA was formed with the objective to set up criteria ensuring protection of the ground water from the point of view that ground water was the main source of drinking water.
The decision criteria were thus based on the Drinking Water Directive and the National Danish Drikkevandsbekendtgørelsen with the limit value specified as 0.1 mg/L for pesticides and pesticide related compounds (for each single compound) and 0.5 mg/L for the sum of compounds.
The fulfilment of these criteria could in principle be assessed in different depths of the soil. In reality, the criteria should be met in the ground water, but it was not possible to make this operational for the registration purpose. For practical reasons it was therefore decided to base the assessment on the annual volume of ground water formed under one field. This definition was considered relevant and in line with other investigations at that time i.e. field and especially lysimeter studies, where an annual volume of leachate was collected and analysed for pesticide and metabolites.
Since lysimeters almost universally were 1 metre deep, it was quite natural to identify 1 metre as a reading point. This was further supported by the fact that field investigations of pesticide movement usually included analysis of soil samples down to approximately 1 metre for quantification of pesticide movement.
At that time, it is important to mention that the conceptual understanding of pesticides leaching was based on matrix transport, only. It was known that leaching could be facilitated by macro pore flow, but this was not included in the assessment as the knowledge about macro pore flow was very sparse especially in relation to the quantitative significance.
Another reason for the selection of 1 metre as a scientifically defendable reading point was that if compounds had leached to a depth of 1 metre further dissipation caused by sorption and degradation was not likely to occur. The assessment was that the concentration of a pesticide would not decline significantly having reached a depth of 1 metre. One argument for this was that the concentration of organic matter, which was thought to be the sole energy resource for the micro-organism, declined strongly with depth meaning that there would be practically no activity under 1 metre. Moreover, the depletion of organic matter was also taken as an indication that adsorption of the compounds would be negligible, as organic matter normally determines the adsorption of pesticides.
It is important to stress that the above-mentioned criteria were only established with regard to the approval system, which by nature is prospective (i.e. it is targeted for pesticides that are not yet on the market). The 1-metre reading point was not meant as a criterion for the assessment of monitoring results. The focus of the approval system regarding the pesticide content in ground water was not a defined depth but one that was definitely larger than 1 meter.
Recently in setting up criteria based on computer simulations of leaching it was a natural extension to use 1 metre as the reading point for the output of simulations. This was necessitated by the wish to be able to make comparisons with lysimeter results. Thus, the development of criteria was an on going parallel process in many countries and consequently the 1 metre reading point was adopted in EU legislation.
Thus, the 1 metre reading point is for practical and protective purposes and do not represent a depth, at which the ground water should comply with the 0.1 mg/L limit.
1.2.2 The European Approach
Principles and interpretations comparable to the Danish approach can be seen in the EU guidance paper on relevant metabolites (European Commission, 2003.), chapter 2: Context and general approach:
“As noted above, this document is intended to provide guidance for the inclusion of active substances in Annex I of Directive 91/414/EEC. According to Art 5 of the Directive “… an active substance shall be included in Annex I … if it may be expected that plant protection products containing the active substance … do not have any harmful effects … on groundwater…”. This possibility of potential groundwater - or drinking water - contamination is investigated generally on the basis of the convention that a soil layer of approximately 1 m is used to represent the “groundwater” aquifer. Such an assumption is far from representative for all regions of Europe but it is considered to provide a realistic worst case on the European scale, in compliance with Art 5 of the Directive. Should, at a future stage, more realistic assessment schemes and models become available for refined assessments at the European scale (e.g. probabilistic assessments based on real groundwater distribution data), this Guidance document will be revised to reflect such a progress.”
Another example on the fact that the 1 metre is a reading point only, can be seen in the report “Generic guidance for FOCUS groundwater scenarios, Version 1.1, April 2002” (FOCUS, 2002), where the executive summary states that “The models all report concentrations at 1m depth for comparative purposes, but this does not represent groundwater”.
Version 1.0 August 2007, © Danish Environmental Protection Agency